Fekete polynomial

In mathematics, a Fekete polynomial is a polynomial

${\displaystyle f_{p}(t):=\sum _{a=0}^{p-1}\left({\frac {a}{p}}\right)t^{a}\,}$

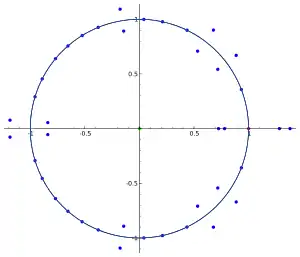
Roots of the Fekete polynomial for p = 43

where ${\displaystyle \left({\frac {\cdot }{p}}\right)\,}$ is the Legendre symbol modulo some integer p > 1.

These polynomials were known in nineteenth-century studies of Dirichlet L-functions, and indeed to Dirichlet himself. They have acquired the name of Michael Fekete, who observed that the absence of real zeroes t of the Fekete polynomial with 0 < t < 1 implies an absence of the same kind for the L-function

${\displaystyle L\left(s,{\dfrac {x}{p}}\right).\,}$

This is of considerable potential interest in number theory, in connection with the hypothetical Siegel zero near s = 1. While numerical results for small cases had indicated that there were few such real zeroes, further analysis reveals that this may indeed be a 'small number' effect.