Entropic gravity
Entropic gravity, also known as emergent gravity, is a theory in modern physics that describes gravity as an entropic force—a force with macro-scale homogeneity but which is subject to quantum-level disorder—and not a fundamental interaction. The theory, based on string theory, black hole physics, and quantum information theory, describes gravity as an emergent phenomenon that springs from the quantum entanglement of small bits of spacetime information. As such, entropic gravity is said to abide by the second law of thermodynamics under which the entropy of a physical system tends to increase over time.
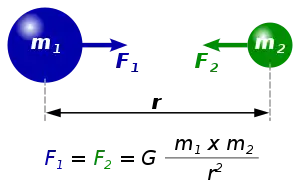
At its simplest, the theory holds that when gravity becomes vanishingly weak—levels seen only at interstellar distances—it diverges from its classically understood nature and its strength begins to decay linearly with distance from a mass.
Entropic gravity provides the underlying framework to explain Modified Newtonian Dynamics, or MOND, which holds that at a gravitational acceleration threshold of approximately 1.2×10−10 m/s2, gravitational strength begins to vary inversely (linearly) with distance from a mass rather than the normal inverse-square law of the distance. This is an exceedingly low threshold, measuring only 12 trillionths gravity's strength at earth's surface; an object dropped from a height of one meter would fall for 36 hours were earth's gravity this weak. It is also 3,000 times less than exists at the point where Voyager 1 crossed our solar system's heliopause and entered interstellar space.
The theory claims to be consistent with both the macro-level observations of Newtonian gravity as well as Einstein's theory of general relativity and its gravitational distortion of spacetime. Importantly, the theory also explains (without invoking the existence of dark matter and its accompanying math featuring new free parameters that are tweaked to obtain the desired outcome) why galactic rotation curves differ from the profile expected with visible matter.
The theory of entropic gravity posits that what has been interpreted as unobserved dark matter is the product of quantum effects that can be regarded as a form of positive dark energy that lifts the vacuum energy of space from its ground state value. A central tenet of the theory is that the positive dark energy leads to a thermal-volume law contribution to entropy that overtakes the area law of anti-de Sitter space precisely at the cosmological horizon.
The theory has been controversial within the physics community but has sparked research and experiments to test its validity.
Origin
The thermodynamic description of gravity has a history that goes back at least to research on black hole thermodynamics by Bekenstein and Hawking in the mid-1970s. These studies suggest a deep connection between gravity and thermodynamics, which describes the behavior of heat. In 1995, Jacobson demonstrated that the Einstein field equations describing relativistic gravitation can be derived by combining general thermodynamic considerations with the equivalence principle.[1] Subsequently, other physicists, most notably Thanu Padmanabhan, began to explore links between gravity and entropy.[2][3]
Erik Verlinde's theory
In 2009, Erik Verlinde proposed a conceptual model that describes gravity as an entropic force.[4] He argues (similar to Jacobson's result) that gravity is a consequence of the "information associated with the positions of material bodies".[5] This model combines the thermodynamic approach to gravity with Gerard 't Hooft's holographic principle. It implies that gravity is not a fundamental interaction, but an emergent phenomenon which arises from the statistical behavior of microscopic degrees of freedom encoded on a holographic screen. The paper drew a variety of responses from the scientific community. Andrew Strominger, a string theorist at Harvard said "Some people have said it can't be right, others that it's right and we already knew it – that it’s right and profound, right and trivial."[6]
In July 2011, Verlinde presented the further development of his ideas in a contribution to the Strings 2011 conference, including an explanation for the origin of dark matter.[7]
Verlinde's article also attracted a large amount of media exposure,[8][9] and led to immediate follow-up work in cosmology,[10][11] the dark energy hypothesis,[12] cosmological acceleration,[13][14] cosmological inflation,[15] and loop quantum gravity.[16] Also, a specific microscopic model has been proposed that indeed leads to entropic gravity emerging at large scales.[17] Entropic gravity can emerge from quantum entanglement of local Rindler horizons.[18]
Derivation of the law of gravitation
The law of gravitation is derived from classical statistical mechanics applied to the holographic principle, that states that the description of a volume of space can be thought of as bits of binary information, encoded on a boundary to that region, a closed surface of area . The information is evenly distributed on the surface with each bit requiring an area equal to , the so-called Planck area, from which can thus be computed:
where is the Planck length. The Planck length is defined as:
where is the universal gravitational constant, is the speed of light, and is the reduced Planck constant. When substituted in the equation for we find:
The statistical equipartition theorem defines the temperature of a system with degrees of freedom in terms of its energy such that:
where is the Boltzmann constant. This is the equivalent energy for a mass according to:
- .
The effective temperature experienced due to a uniform acceleration in a vacuum field according to the Unruh effect is:
- ,
where is that acceleration, which for a mass would be attributed to a force according to Newton's second law of motion:
- .
Taking the holographic screen to be a sphere of radius , the surface area would be given by:
- .
From algebraic substitution of these into the above relations, one derives Newton's law of universal gravitation:
- .
Note that this derivation assumes that the number of the binary bits of information is equal to the number of the degrees of freedom.
Criticism and experimental tests
Entropic gravity, as proposed by Verlinde in his original article, reproduces the Einstein field equations and, in a Newtonian approximation, a 1/r potential for gravitational forces. Since its results do not differ from Newtonian gravity except in regions of extremely small gravitational fields, testing the theory with earth-based laboratory experiments does not appear feasible. Spacecraft-based experiments performed at Lagrangian points within our solar system would be expensive and challenging.
Even so, entropic gravity in its current form has been severely challenged on formal grounds. Matt Visser has shown[19] that the attempt to model conservative forces in the general Newtonian case (i.e. for arbitrary potentials and an unlimited number of discrete masses) leads to unphysical requirements for the required entropy and involves an unnatural number of temperature baths of differing temperatures. Visser concludes:
There is no reasonable doubt concerning the physical reality of entropic forces, and no reasonable doubt that classical (and semi-classical) general relativity is closely related to thermodynamics [52–55]. Based on the work of Jacobson [1–6], Thanu Padmanabhan [7–12], and others, there are also good reasons to suspect a thermodynamic interpretation of the fully relativistic Einstein equations might be possible. Whether the specific proposals of Verlinde [26] are anywhere near as fundamental is yet to be seen – the rather baroque construction needed to accurately reproduce n-body Newtonian gravity in a Verlinde-like setting certainly gives one pause.
For the derivation of Einstein's equations from an entropic gravity perspective, Tower Wang shows[20] that the inclusion of energy-momentum conservation and cosmological homogeneity and isotropy requirements severely restrict a wide class of potential modifications of entropic gravity, some of which have been used to generalize entropic gravity beyond the singular case of an entropic model of Einstein's equations. Wang asserts that:
As indicated by our results, the modified entropic gravity models of form (2), if not killed, should live in a very narrow room to assure the energy-momentum conservation and to accommodate a homogeneous isotropic universe.
Cosmological observations using available technology can be used to test the theory. On the basis of lensing by the galaxy cluster Abell 1689, Nieuwenhuizen concludes that EG is strongly ruled out unless additional (dark) matter like eV neutrinos is added.[21] A team from Leiden Observatory statistically observing the lensing effect of gravitational fields at large distances from the centers of more than 33,000 galaxies, found that those gravitational fields were consistent with Verlinde's theory.[22][23][24] Using conventional gravitational theory, the fields implied by these observations (as well as from measured galaxy rotation curves) could only be ascribed to a particular distribution of dark matter. In June 2017, a study by Princeton University researcher Kris Pardo asserted that Verlinde's theory is inconsistent with the observed rotation velocities of dwarf galaxies.[25][26]
Sabine Hossenfelder argues that "one should interpret these studies [comparing dark matter gravitational studies with EG] with caution" because "approximations must be made to arrive at [the to be tested EG] equation[s]" and it's not yet clear that the approximations are themselves correct.[27]
In 2018, Zhi-Wei Wang and Samuel L. Braunstein showed that, while spacetime surfaces near black holes (called stretched horizons) do obey an analog of the first law of thermodynamics, ordinary spacetime surfaces — including holographic screens — generally do not, thus undermining the key thermodynamic assumption of the emergent gravity program.[28]
Entropic gravity and quantum coherence
Another criticism of entropic gravity is that entropic processes should, as critics argue, break quantum coherence. There is no theoretical framework quantitatively describing the strength of such decoherence effects, though. The temperature of the gravitational field in earth gravity well is very small (on the order of 10−19K).
Experiments with ultra-cold neutrons in the gravitational field of Earth are claimed to show that neutrons lie on discrete levels exactly as predicted by the Schrödinger equation considering the gravitation to be a conservative potential field without any decoherent factors. Archil Kobakhidze argues that this result disproves entropic gravity,[29] while Chaichian et al. suggest a potential loophole in the argument in weak gravitational fields such as those affecting Earth-bound experiments.[30]
See also
- Abraham–Lorentz force
- Beyond black holes – Area of physical study that seeks to reconcile the laws of thermodynamics with the existence of black hole event horizons
- Black hole electron
- Entropic force
- Hawking radiation – Black-hole radiation predicted by Stephen Hawking
- List of quantum gravity researchers – Wikipedia list article
- Entropic elasticity of an ideal chain
- Gravity – Phenomenon of attraction between objects with mass
- Induced gravity
References
- Jacobson, Theodore (4 April 1995). "Thermodynamics of Spacetime: The Einstein Equation of State". Phys. Rev. Lett. 75 (7): 1260–1263. arXiv:gr-qc/9504004. Bibcode:1995PhRvL..75.1260J. doi:10.1103/PhysRevLett.75.1260. PMID 10060248. S2CID 13223728.
- Padmanabhan, Thanu (2010). "Thermodynamical Aspects of Gravity: New insights". Rep. Prog. Phys. 73 (4): 6901. arXiv:0911.5004. Bibcode:2010RPPh...73d6901P. doi:10.1088/0034-4885/73/4/046901.
- Mok, H.M. (13 August 2004). "Further Explanation to the Cosmological Constant Problem by Discrete Space-time Through Modified Holographic Principle". arXiv:physics/0408060.
- van Calmthout, Martijn (12 December 2009). "Is Einstein een beetje achterhaald?". de Volkskrant (in Dutch). Retrieved 6 September 2010.
- E.P. Verlinde (2011). "On the Origin of Gravity and the Laws of Newton". JHEP. 2011 (4): 29. arXiv:1001.0785. Bibcode:2011JHEP...04..029V. doi:10.1007/JHEP04(2011)029. S2CID 3597565.
- Overbye, Dennis (12 July 2010). "A Scientist Takes on Gravity". The New York Times. Retrieved 6 September 2010.
- E. Verlinde, The Hidden Phase Space of our Universe, Strings 2011, Uppsala, 1 July 2011.
- The entropy force: a new direction for gravity, New Scientist, 20 January 2010, issue 2744
- Gravity is an entropic form of holographic information, Wired Magazine, 20 January 2010
- Fu-Wen Shu; Yungui Gong (2011). "Equipartition of energy and the first law of thermodynamics at the apparent horizon". International Journal of Modern Physics D. 20 (4): 553–559. arXiv:1001.3237. Bibcode:2011IJMPD..20..553S. doi:10.1142/S0218271811018883. S2CID 119253807.
- Rong-Gen Cai; Li-Ming Cao; Nobuyoshi Ohta (2010). "Friedmann Equations from Entropic Force". Phys. Rev. D. 81 (6): 061501. arXiv:1001.3470. Bibcode:2010PhRvD..81f1501C. CiteSeerX 10.1.1.756.6761. doi:10.1103/PhysRevD.81.061501. S2CID 118462566.
- It from Bit: How to get rid of dark energy, Johannes Koelman, 2010
- Easson; Frampton; Smoot (2011). "Entropic Accelerating Universe". Phys. Lett. B. 696 (3): 273–277. arXiv:1002.4278. Bibcode:2011PhLB..696..273E. doi:10.1016/j.physletb.2010.12.025. S2CID 119192004.
- Yi-Fu Cai; Jie Liu; Hong Li (2010). "Entropic cosmology: a unified model of inflation and late-time acceleration". Phys. Lett. B. 690 (3): 213–219. arXiv:1003.4526. Bibcode:2010PhLB..690..213C. doi:10.1016/j.physletb.2010.05.033. S2CID 118627323.
- Yi Wang (2010). "Towards a Holographic Description of Inflation and Generation of Fluctuations from Thermodynamics". arXiv:1001.4786 [hep-th].
- Lee Smolin (2010). "Newtonian gravity in loop quantum gravity". arXiv:1001.3668 [gr-qc].
- Jarmo Mäkelä (2010). "Notes Concerning "On the Origin of Gravity and the Laws of Newton" by E. Verlinde". arXiv:1001.3808 [gr-qc].
- Lee, Jae-Weon; Kim, Hyeong-Chan; Lee, Jungjai (2013). "Gravity from quantum information". Journal of the Korean Physical Society. 63 (5): 1094–1098. arXiv:1001.5445. Bibcode:2013JKPS...63.1094L. doi:10.3938/jkps.63.1094. ISSN 0374-4884. S2CID 118494859.
- Visser, Matt (2011). "Conservative entropic forces". JHEP. 1110 (10): 140. arXiv:1108.5240. Bibcode:2011JHEP...10..140V. doi:10.1007/JHEP10(2011)140. S2CID 119097091., to appear in JHEP
- Wang, Tower (2012). "Modified entropic gravity revisited". arXiv:1211.5722 [hep-th].
- Nieuwenhuizen, Theodorus M. (5 October 2016). "How Zwicky already ruled out modified gravity theories without dark matter". Fortschritte der Physik. 65 (6–8): 1600050. arXiv:1610.01543. doi:10.1002/prop.201600050. S2CID 118676940.
- "Verlinde's new theory of gravity passes first test". 16 December 2016.
- Brouwer, Margot M.; et al. (11 December 2016). "First test of Verlinde's theory of Emergent Gravity using Weak Gravitational Lensing measurements". Monthly Notices of the Royal Astronomical Society. 466 (to appear): 2547–2559. arXiv:1612.03034. Bibcode:2017MNRAS.466.2547B. doi:10.1093/mnras/stw3192. S2CID 18916375.
- "First test of rival to Einstein's gravity kills off dark matter". 15 December 2016. Retrieved 20 February 2017.
- "Researchers Check Space-Time to See if It's Made of Quantum Bits". Quanta Magazine. 21 June 2017. Retrieved 11 August 2017.
Emergent gravity successfully predicts the rotation velocities of the smallest galaxies in the sample. But it predicts velocities far too low for the more massive galaxies, especially the ones full of gas clouds. This discrepancy could pose a serious problem for emergent gravity, since the main success of the theory so far has been predicting the rotation curves of large galaxies.
- Pardo, Kris (2 June 2017). Testing Emergent Gravity with Isolated Dwarf Galaxies (Report). arXiv:1706.00785. Bibcode:2017arXiv170600785P. (PDF). Accessed 2017-06-22.
- Hossenfelder, Sabine (28 February 2017). "Recent Claims Invalid: Emergent Gravity Might Deliver A Universe Without Dark Matter". forbes.com. Forbes. Retrieved 18 July 2018.
- Wang, Zhi-Wei; Braunstein, Samuel L. (2018). "Surfaces away from horizons are not thermodynamic". Nature Communications. 9 (1): 2977. doi:10.1038/s41467-018-05433-9. PMC 6065406. PMID 30061720.
- Kobakhidze, Archil (2011). "Gravity is not an entropic force". Physical Review D. arXiv:1009.5414.
- Chaichian, M.; Oksanen, M.; Tureanu, A. (2011). "On gravity as an entropic force". Physics Letters B. 702 (5): 419–421. arXiv:1104.4650. Bibcode:2011PhLB..702..419C. doi:10.1016/j.physletb.2011.07.019. S2CID 119287340.