Koide formula
The Koide formula is an unexplained empirical equation discovered by Yoshio Koide in 1981. In its original form, it relates the masses of the three charged leptons; later authors have extended the relation to neutrinos, quarks, and other families of particles.[1]:64–66
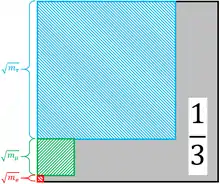
Formula
The Koide formula is
where the masses of the electron, muon, and tau are measured respectively as me = 0.510998946(3) MeV/c2, mμ = 105.6583745(24) MeV/c2, and mτ = 1776.86(12) MeV/c2; the digits in parentheses are the uncertainties in the last figures.[2] This gives Q = 0.666661(7).[3]
No matter what masses are chosen to stand in place of the electron, muon, and tau, 1/3 ≤ Q < 1. The upper bound follows from the fact that the square roots are necessarily positive, and the lower bound follows from the Cauchy–Bunyakovsky–Schwarz inequality. The experimentally determined value, 2/3, lies at the center of the mathematically allowed range.
The mystery is in the physical value. Not only is the result peculiar, in that three ostensibly arbitrary numbers give a simple fraction, but also in that in the case of electron, muon, and tau, Q is exactly halfway between the two extremes of all possible combinations: 1/3 (if the three masses were equal) and 1 (if one mass dominates).
The Koide formula can also be interpreted as geometrical relation, in which the value is the squared cosine of the angle between the vector and the vector (see dot product).[4] That angle is almost exactly 45 degrees: .[4]
When the formula is assumed to hold exactly (Q = 2/3) it may be used to predict the tau mass from the (more precisely known) electron and muon masses; that prediction is mτ = 1776.969 MeV/c2.[5]
While the original formula arose in the context of preon models, other ways have been found to derive it (both by Sumino and by Koide – see references below). As a whole, however, understanding remains incomplete. Similar matches have been found for triplets of quarks depending on running masses.[6][7][8] With alternating quarks, chaining Koide equations for consecutive triplets, it is possible to reach a result of 173.263947(6) GeV for the mass of the top quark.[9]
Speculative extension
It has been proposed[5] that the lepton masses are given by the squares of the eigenvalues of a circulant matrix with real eigenvalues, corresponding to the relation
which can be fit to experimental data with η2 = 0.500003(23) (corresponding to the Koide relation), and phase δ = 0.2222220(19), which is almost exactly 2/9. However, the experimental data are in conflict with simultaneous equality of η2 = 1/2 and δ = 2/9.[5]
This kind of relation has also been proposed for the quark families, with phases equal to low-energy values 2/27 and 4/27, hinting at a relation with the charge of the particle family (1/3 and 2/3 for quarks vs. 1 for the leptons).[10]
Similar formulae
There are similar empirical formulae which relate other masses. Quark masses depend on the energy scale used to measure them, which makes an analysis more complicated.[11]:147
Taking the heaviest three quarks, charm (1.275 ± 0.03 GeV), bottom (4.180 ± 0.04 GeV) and top (173.0 ± 0.40 GeV), and without using their uncertainties gives the value cited by F. G. Cao (2012),[12]
This was noticed by Rodejohann and Zhang in the first version of their 2011 article[13] but the observation was removed in the published version,[6] so the first published mention is in 2012 from Cao.[12]
Similarly, the masses of the lightest quarks, up (2.2 ± 0.4 MeV), down (4.7 ± 0.3 MeV), and strange (95.0 ± 4.0 MeV), without using their experimental uncertainties yield,
a value also cited by Cao in the same paper.[12]
Running of particle masses
In quantum field theory, quantities like coupling constant and mass "run" with the energy scale. That is, their value depends on the energy scale at which the observation occurs, in a way described by a renormalization group equation (RGE).[14] One usually expects relationships between such quantities to be simple at high energies (where some symmetry is unbroken) but not at low energies, where the RG flow will have produced complicated deviations from the high-energy relation. The Koide relation is exact (within experimental error) for the pole masses, which are low-energy quantities defined at different energy scales. For this reason, many physicists regard the relation as "numerology".[15]
However, the Japanese physicist Yukinari Sumino has proposed mechanisms to explain origins of the charged lepton spectrum as well as the Koide formula, e.g., by constructing an effective field theory in which a new gauge symmetry causes the pole masses to exactly satisfy the relation.[16] Koide has published his opinions concerning Sumino's model.[17][18] François Goffinet's doctoral thesis gives a discussion on pole masses and how the Koide formula can be reformulated without taking the square roots of masses.[19]
See also
References
- Zenczykowski, P., Elementary Particles And Emergent Phase Space (Singapore: World Scientific, 2014), pp. 64–66.
- Amsler, C.; et al. (Particle Data Group) (2008). "Review of Particle Physics" (PDF). Physics Letters B. 667 (1–5): 1–6. Bibcode:2008PhLB..667....1A. doi:10.1016/j.physletb.2008.07.018. PMID 10020536.
- Since the uncertainties in me and mμ are much smaller than e that in mτ, the uncertainty in Q was calculated as .
- Foot, R. (1994-02-07). "A note on Koide's lepton mass relation". arXiv:hep-ph/9402242.
- Brannen, Carl A. (May 2, 2006). "The Lepton Masses" (PDF). Brannen's personal website. Retrieved 18 Oct 2020.
- Rodejohann, W.; Zhang, H. (2011). "Extension of an empirical charged lepton mass relation to the neutrino sector". Physics Letters B. 698 (2): 152–156. arXiv:1101.5525. Bibcode:2011PhLB..698..152R. doi:10.1016/j.physletb.2011.03.007. S2CID 59445811.
- Rosen, G. (2007). "Heuristic development of a Dirac-Goldhaber model for lepton and quark structure" (PDF). Modern Physics Letters A. 22 (4): 283–288. Bibcode:2007MPLA...22..283R. doi:10.1142/S0217732307022621.
- Kartavtsev, A. (2011). "A remark on the Koide relation for quarks". arXiv:1111.0480 [hep-ph].
- Rivero, A. (2011). "A new Koide tuple: Strange-charm-bottom". arXiv:1111.7232 [hep-ph].
- Zenczykowski, Piotr (2012-12-26). "Remark on Koide's Z3-symmetric parametrization of quark masses". Physical Review D. 86 (11): 117303. arXiv:1210.4125. Bibcode:2012PhRvD..86k7303Z. doi:10.1103/PhysRevD.86.117303. ISSN 1550-7998. S2CID 119189170.
- Quadt, A., Top Quark Physics at Hadron Colliders (Berlin/Heidelberg: Springer, 2006), p. 147.
- Cao, F. G. (2012). "Neutrino masses from lepton and quark mass relations and neutrino oscillations". Physical Review D. 85 (11): 113003. arXiv:1205.4068. Bibcode:2012PhRvD..85k3003C. doi:10.1103/PhysRevD.85.113003. S2CID 118565032.
- Rodejohann, W.; Zhang, H. (2011). "Extension of an empirical charged lepton mass relation to the neutrino sector". arXiv:1101.5525 [hep-ph].
- Green, D., Cosmology with MATLAB (Singapore: World Scientific, 2016), p. 197.
- Motl, L. (16 January 2012). "Could the Koide formula be real?". The Reference Frame. Retrieved 2014-07-10.
- Sumino, Y. (2009). "Family Gauge Symmetry as an Origin of Koide's Mass Formula and Charged Lepton Spectrum". Journal of High Energy Physics. 2009 (5): 75. arXiv:0812.2103. Bibcode:2009JHEP...05..075S. doi:10.1088/1126-6708/2009/05/075. S2CID 14238049.
- Koide, Yoshio (2017). "Sumino Model and My Personal View". arXiv:1701.01921 [hep-ph].
- Koide, Yoshio (2018). "What Physics Does The Charged Lepton Mass Relation Tell Us?". arXiv:1809.00425 [hep-ph].
- Goffinet, F. (2008). A bottom-up approach to fermion masses (PDF) (PhD Thesis). Université catholique de Louvain.
Further reading
- Koide, Y. (1983). "New view of quark and lepton mass hierarchy". Physical Review D. 28 (1): 252–254. Bibcode:1983PhRvD..28..252K. doi:10.1103/PhysRevD.28.252.
- Koide, Y. (1984). "Erratum: New view of quark and lepton mass hierarchy". Physical Review D. 29 (7): 1544. Bibcode:1984PhRvD..29Q1544K. doi:10.1103/PhysRevD.29.1544.
- Koide, Y. (1983). "A fermion-boson composite model of quarks and leptons". Physics Letters B. 120 (1–3): 161–165. Bibcode:1983PhLB..120..161K. doi:10.1016/0370-2693(83)90644-5.
- Oneda, S.; Koide, Y. (1991). Asymptotic symmetry and its implication in elementary particle physics. World Scientific. ISBN 978-981-02-0498-3.
- Foot, R. (1994). "A note on Koide's lepton mass relation". arXiv:hep-ph/9402242.
- Koide, Y. (2000). "Quark and lepton mass matrices with a cyclic permutation invariant form". arXiv:hep-ph/0005137.
- Rivero, A.; Gsponer, A. (2005). "The strange formula of Dr. Koide". arXiv:hep-ph/0505220.
- Koide, Y. (2005). "Challenge to the mystery of the charged lepton mass". arXiv:hep-ph/0506247.
- Li, N.; Ma, B.-Q. (2006). "Energy scale independence for quark and lepton masses". Physical Review D. 73 (1): 013009. arXiv:hep-ph/0601031. Bibcode:2006PhRvD..73a3009L. doi:10.1103/PhysRevD.73.013009. S2CID 2624370.
- Brannen, C. (2010). "Spin Path Integrals and Generations" (PDF). Foundations of Physics. 40 (11): 1681–1699. arXiv:1006.3114. Bibcode:2010FoPh...40.1681B. CiteSeerX 10.1.1.749.3756. doi:10.1007/s10701-010-9465-8. S2CID 11007648. (See the article's references links to "The lepton masses" and "Recent results from the MINOS experiment".)
- Kocik, J. (2012). "The Koide lepton mass formula and geometry of circles". arXiv:1201.2067 [physics.gen-ph].
External links
- Wolfram Alpha, link solves for the predicted tau mass from the Koide formula