Trace operator
In mathematics, the trace operator extends the notion of the restriction of a function to the boundary of its domain to "generalized" functions in a Sobolev space. This is particularly important for the study of partial differential equations with prescribed boundary conditions (boundary value problems), where weak solutions may not be regular enough to satisfy the boundary conditions in the classical sense of functions.
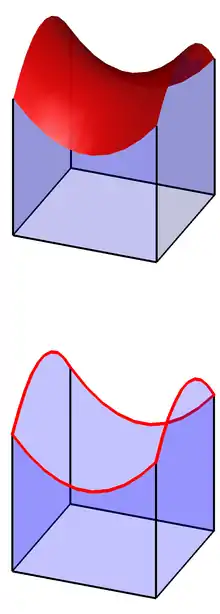
Motivation
On a bounded, smooth domain , consider the problem of solving Poisson's equation with inhomogeneous Dirichlet boundary conditions:
with given functions and with regularity discussed in the application section below. The weak solution of this equation must satisfy
- for all .
The -regularity of is sufficient for the well-definedness of this integral equation. It is not apparent, however, in which sense can satisfy the boundary condition on : by definition, is an equivalence class of functions which can have arbitrary values on since this is a null set with respect to the n-dimensional Lebesgue measure.
If there holds by Sobolev's embedding theorem, such that can satisfy the boundary condition in the classical sense, i.e. the restriction of to agrees with the function (more precisely: there exists a representative of in with this property). For with such an embedding does not exist and the trace operator presented here must be used to give meaning to . Then with is called a weak solution to the boundary value problem if the integral equation above is satisfied. For the definition of the trace operator to be reasonable, there must hold for sufficiently regular .
Trace theorem
The trace operator can be defined for functions in the Sobolev spaces with , see the section below for possible extensions of the trace to other spaces. Let for be a bounded domain with Lipschitz boundary. Then[1] there exists a bounded linear trace operator
such that extends the classical trace, i.e.
- for all .
The continuity of implies that
- for all
with constant only depending on and . The function is called trace of and is often simply denoted by . Other common symbols for include and .
Construction
This paragraph follows Evans,[2] where more details can be found, and assumes that has a -boundary. A proof (of a stronger version) of the trace theorem for Lipschitz domains can be found in Gagliardo.[1] On a -domain, the trace operator can be defined as continuous linear extension of the operator
to the space . By density of in such an extension is possible if is continuous with respect to the -norm. The proof of this, i.e. that there exists (depending on and ) such that
- for all
is the central ingredient in the construction of the trace operator. A local variant of this estimate for -functions is first proven for a locally flat boundary using the divergence theorem. By transformation, a general -boundary can by locally straightened to reduce to this case, where the -regularity of the transformation requires that the local estimate holds for -functions.
With this continuity of the trace operator in an extension to exists by abstract arguments and for can be characterized as follows. Let be a sequence approximating by density. By the proven continuity of in the sequence is a Cauchy sequence in and with limit taken in .
The extension property holds for by construction, but for any there exists a sequence which converges uniformly on to , verifying the extension property on the larger set .
The case p = ∞
If is bounded and has a -boundary then by Morrey's inequality there exists a continuous embedding , where denotes the space of Lipschitz continuous functions. In particular, any function has a classical trace and there holds
Functions with trace zero
The Sobolev spaces for are defined as the closure of the set of compactly supported test functions with respect to the -norm. The following alternative characterization holds:
where is the kernel of , i.e. is the subspace of functions in with trace zero.
Image of the trace operator
For p > 1
The trace operator is not surjective onto if , i.e. not every function in is the trace of a function in . As elaborated below the image consists of functions which satisfy a -version of Hölder continuity.
Abstract characterization
An abstract characterization of the image of can be derived as follows. By the isomorphism theorems there holds
where denotes the quotient space of the Banach space by the subspace and the last identity follows from the characterization of from above. Equipping the quotient space with the quotient norm defined by
the trace operator is then a surjective, bounded linear operator
- .
Characterization using Sobolev–Slobodeckij spaces
A more concrete representation of the image of can be given using Sobolev-Slobodeckij spaces which generalize the concept of Hölder continuous functions to the -setting. Since is a (n-1)-dimensional Lipschitz manifold embedded into an explicit characterization of these spaces is technically involved. For simplicity consider first a planar domain . For define the (possibly infinite) norm
which generalizes the Hölder condition . Then
equipped with the previous norm is a Banach space (a general definition of for non-integer can be found in the article for Sobolev-Slobodeckij spaces). For the (n-1)-dimensional Lipschitz manifold define by locally straightening and proceeding as in the definition of .
The space can then be identified as the image of the trace operator and there holds[1] that
is a surjective, bounded linear operator.
For p = 1
For the image of the trace operator is and there holds[1] that
is a surjective, bounded linear operator.
Right-inverse: trace extension operator
The trace operator is not injective since multiple functions in can have the same trace (or equivalently, ). The trace operator has however a well-behaved right-inverse, which extends a function defined on the boundary to the whole domain. Specifically, for there exists a bounded, linear trace extension operator[3]
- ,
using the Sobolev-Slobodeckij characterization of the trace operator's image from the previous section, such that
- for all
and, by continuity, there exists with
- .
Notable is not the mere existence but the linearity and continuity of the right inverse. This trace extension operator must not be confused with the whole-space extension operators which play a fundamental role in the theory of Sobolev spaces.
Extension to other spaces
Higher derivatives
Many of the previous results can be extended to with higher differentiability if the domain is sufficiently regular. Let denote the exterior unit normal field on . Since can encode differentiability properties in tangential direction only the normal derivative is of additional interest for the trace theory for . Similar arguments apply to higher-order derivatives for .
Let and be a bounded domain with -boundary. Then[3] there exists a surjective, bounded linear higher-order trace operator
with Sobolev-Slobodeckij spaces for non-integer defined on through transformation to the planar case for , whose definition is elaborated in the article on Sobolev-Slobodeckij spaces. The operator extends the classical normal traces in the sense that
- for all
Furthermore, there exists a bounded, linear right-inverse of , a higher-order trace extension operator[3]
- .
Finally, the spaces , the completion of in the -norm, can be characterized as the kernel of ,[3] i.e.
- .
No trace in Lp
There is no sensible extension of the concept of traces to for since any bounded linear operator which extends the classical trace must be zero on the space of test functions , which is a dense subset of , implying that such an operator would be zero everywhere.
Generalized normal trace
Let denote the distributional divergence of a vector field . For and bounded Lipschitz domain define
which is a Banach space with norm
- .
Let denote the exterior unit normal field on . Then[4] there exists a bounded linear operator
- ,
where is the conjugate exponent to and denotes the continuous dual space to a Banach space , such that extends the normal trace for in the sense that
- .
The value of the normal trace operator for is defined by application of the divergence theorem to the vector field where is the trace extension operator from above.
Application. Any weak solution to in a bounded Lipschitz domain has a normal derivative in the sense of . This follows as since and . This result is notable since in Lipschitz domains in general , such that may not lie in the domain of the trace operator .
Application
The theorems presented above allow a closer investigation of the boundary value problem
on a Lipschitz domain from the motivation. Since only the Hilbert space case is investigated here, the notation is used to denote etc. As stated in the motivation, a weak solution to this equation must satisfy and
- for all ,
where the right-hand side must be interpreted for as a duality product with the value .
Existence and uniqueness of weak solutions
The characterization of the range of implies that for to hold the regularity is necessary. This regularity is also sufficient for the existence of a weak solution, which can be seen as follows. By the trace extension theorem there exists such that . Defining by we have that and thus by the characterization of as space of trace zero. The function then satisfies the integral equation
- for all .
Thus the problem with inhomogeneous boundary values for could be reduced to a problem with homogeneous boundary values for , a technique which can be applied to any linear differential equation. By the Riesz representation theorem there exists a unique solution to this problem. By uniqueness of the decomposition , this is equivalent to the existence of a unique weak solution to the inhomogeneous boundary value problem.
Continuous dependence on the data
It remains to investigate the dependence of on and . Let denote constants independent of and . By continuous dependence of on the right-hand side of its integral equation, there holds
and thus, using that and by continuity of the trace extension operator, it follows that
and the solution map
is therefore continuous.
References
- Gagliardo, Emilio (1957). "Caratterizzazioni delle tracce sulla frontiera relative ad alcune classi di funzioni in n variabili". Rendiconti del Seminario Matematico della Università di Padova. 27: 284–305.
- Evans, Lawrence (1998). Partial differential equations. Providence, R.I.: American Mathematical Society. pp. 257–261. ISBN 0-8218-0772-2.
- Nečas, Jindřich (1967). Les méthodes directes en théorie des équations elliptiques. Paris: Masson et Cie, Éditeurs, Prague: Academia, Éditeurs. pp. 90–104.
- Sohr, Hermann (2001). The Navier-Stokes Equations: An Elementary Functional Analytic Approach. Basel: Birkhäuser. pp. 50–51. doi:10.1007/978-3-0348-8255-2.
- Leoni, Giovanni (2017). A First Course in Sobolev Spaces: Second Edition. Graduate Studies in Mathematics. 181. American Mathematical Society. pp. 734. ISBN 978-1-4704-2921-8
