Angular unit
Throughout history, angles have been measured in many different units. These are known as angular units, with the most contemporary units being the degree ( ° ), the radian (rad), and the gradian (grad), though many others have been used throughout history.[1][2] The purpose of this page is to aggregate other concepts pertaining to the angular unit, where additional explanation can be provided.
Angle measurement in general
The size of a geometric angle is usually characterized by the magnitude of the smallest rotation that maps one of the rays into the other. Angles that have the same size are said to be equal, congruent, or equal in measure.
In some contexts, such as identifying a point on a circle or describing the orientation of an object in two dimensions relative to a reference orientation, angles that differ by an exact multiple of a full turn are effectively equivalent. In other contexts, such as identifying a point on a spiral curve, or describing the cumulative rotation of an object in two dimensions relative to a reference orientation, angles that differ by a non-zero multiple of a full turn are not equivalent.
In order to measure an angle θ, a circular arc centered at the vertex of the angle is drawn (e.g. with a pair of compasses). The ratio of the length s of the arc by the radius r of the circle is the measure of the angle in radians.
The measure of the angle in another angular unit is then obtained by multiplying its measure in radians by the scaling factor k/2π, where k is the measure of a complete turn in the chosen unit (for example, 360 for degrees or 400 for gradians):
The value of θ thus defined is independent of the size of the circle: if the length of the radius is changed, then the arc length changes in the same proportion, so the ratio s/r is unaltered. (Proof. The formula above can be rewritten as k = θr/s. One turn, for which θ = n units, corresponds to an arc equal in length to the circle's circumference, which is 2πr, so s = 2πr. Substituting n for θ and 2πr for s in the formula, results in k = nr/2πr = n/2π.) [nb 1]
In particular, the measure of angle is radian can be also interpreted as the arc length of its corresponding unit circle:[4]
Angle addition postulate
The angle addition postulate states that if B is in the interior of angle AOC, then
The measure of the angle AOC is the sum of the measure of angle AOB and the measure of angle BOC. In this postulate, it does not matter in which unit the angle is measured—as long as each angle is measured in the same unit.
Units
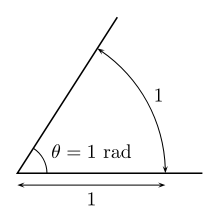
One radian is the angle subtended by an arc of a circle that has the same length as the circle's radius. The radian is the derived quantity of angular measurement in the SI system. By definition, it is dimensionless, though it may be specified as rad to avoid ambiguity. Angles measured in degrees, are shown with the symbol °. Subdivisions of the degree are minute (symbol ′, 1′ = 1/60°) and second {symbol ″, 1″ = 1/3600°}.[1] An angle of 360° corresponds to the angle subtended by a full circle, and is equal to 2π radians, or 400 gradians.
Other units used to represent angles are listed in the following table. These units are defined such that the number of turns is equivalent to a full circle.
| name | turns | rotation angle | description |
|---|---|---|---|
| Turn | 1 | 360° | The turn, also cycle, full circle, revolution, and rotation, is complete circular movement or measure (as to return to the same point) with circle or ellipse. A turn is abbreviated τ, cyc, rev, or rot depending on the application.. The symbol τ can also be used as a mathematical constant to represent 2π radians. |
| Multiples of π | 2 | 180° | The multiples of π (MULπ) unit is implemented in the RPN scientific calculator WP 43S.[5][6][7] See also: IEEE 754 recommended operations |
| Quadrant | 4 | 90° | One quadrant is also known as a right angle. The quadrant is the unit used in Euclid's Elements. In German the symbol ∟ has been used to denote a quadrant. |
| Sextant | 6 | 60° | The sextant was the unit used by the Babylonians,[8][9] The degree, minute of arc and second of arc are sexagesimal subunits of the Babylonian unit. It is especially easy to construct with ruler and compasses. |
| Radian | 2π | 57°17′ | The radian is determined by the circumference of a circle that is equal in length to the radius of the circle. |
| Hexacontade | 60 | 6° | The hexacontade is a unit used by Eratosthenes. |
| Binary degree | 256 | 1°33'45" | The binary degree, also known as the binary radian (or brad).[10] The binary degree is used in computing so that an angle can be efficiently represented in a single byte (albeit to limited precision). Other measures of angle used in computing may be based on dividing one whole turn into 2n equal parts for other values of n. |
| Degree | 360 | 1° | One advantage of this old sexagesimal subunit is that many angles common in simple geometry are measured as a whole number of degrees. Fractions of a degree may be written in normal decimal notation (e.g. 3.5° for three and a half degrees), but the "minute" and "second" sexagesimal subunits of the "degree-minute-second" system are also in use, especially for geographical coordinates and in astronomy and ballistics |
| Grad | 400 | 0°54′ | The grad, also called grade, gradian, or gon. a right angle is 100 grads. It is a decimal subunit of the quadrant. A kilometre was historically defined as a centi-grad of arc along a meridian of the Earth, so the kilometer is the decimal analog to the sexagesimal nautical mile. The grad is used mostly in triangulation. |
| Minute of arc | 21,600 | 0°1′ | The minute of arc (or MOA, arcminute, or just minute) is 1/60 of a degree. A nautical mile was historically defined as a minute of arc along a great circle of the Earth. |
| Second of arc | 1,296,000 | 0°0′1″ | The second of arc (or arcsecond, or just second) is 1/60 of a minute of arc and 1/3600 of a degree. |
Equivalent time descriptors
In astronomy, right ascension and declination are usually measured in angular units, expressed in terms of time, based on a 24-hour day.
| Unit | Symbol | Degree | Radians | Circle | Other |
|---|---|---|---|---|---|
| Hour | h | 15° | π⁄12 | 1⁄24 | |
| Minute | m | 0°15' | π⁄720 | 1⁄1,440 | 1⁄60 hour |
| Second | s | 0°0'15" | π⁄43200 | 1⁄86,400 | 1⁄60 minute |
Other descriptors
- Tau, the number of radians in one turn (1 turn = τ rad), τ = 2π.
- Chi, an old Chinese angle measurement.
- Diameter part (n = 376.99...): The diameter part (occasionally used in Islamic mathematics) is 1/60 radian. One "diameter part" is approximately 0.95493°. There are about 376.991 diameter parts per turn.
- Milliradian and derived definitions: The true milliradian is defined a thousandth of a radian, which means that a rotation of one turn would equal exactly 2000π mil (or approximately 6283.185 mil), and almost all scope sights for firearms are calibrated to this definition. In addition there are three other derived definitions used for artillery and navigation which are approximately equal to a milliradian. Under these three other definitions one turn makes up for exactly 6000, 6300 or 6400 mils, which equals spanning the range from 0.05625 to 0.06 degrees (3.375 to 3.6 minutes). In comparison, the true milliradian is approximately 0.05729578 degrees (3.43775 minutes). One "NATO mil" is defined as 1/6400 of a circle. Just like with the true milliradian, each of the other definitions exploits the mil's useful property of subtensions, i.e. that the value of one milliradian approximately equals the angle subtended by a width of 1 meter as seen from 1 km away (2π/6400 = 0.0009817… ≈ 1/1000).
- Akhnam and zam. In old Arabia a turn was subdivided in 32 Akhnam and each akhnam was subdivided in 7 zam, so that a turn is 224 zam.
Positive and negative angles
Although the definition of the measurement of an angle does not support the concept of a negative angle, it is frequently useful to impose a convention that allows positive and negative angular values to represent orientations, and rotations in opposite directions relative to some reference.
In a two-dimensional Cartesian coordinate system, an angle is typically defined by its two sides, with its vertex at the origin. The initial side is on the positive x-axis, while the other side (i.e., the terminal side) is defined by the measure from the initial side in radians, degrees, or turns. Typically, positive angles represent rotations toward the positive y-axis, and negative angles represent rotations toward the negative y-axis. When Cartesian coordinates are represented by standard position, defined by the x-axis rightward and the y-axis upward, positive rotations are anticlockwise, and negative rotations are clockwise.
In many contexts, an angle of −θ is effectively equivalent to an angle of "one full turn minus θ". For example, an orientation represented as −45° is effectively equivalent to an orientation represented as 360° − 45° or 315°. However, a rotation of −45° would not be the same as a rotation of 315°.
In three-dimensional geometry, "clockwise" and "anticlockwise" have no absolute meaning, so the direction of positive and negative angles must be defined relative to some reference, which is typically a vector passing through the angle's vertex, and which is perpendicular to the plane in which the rays of the angle lie.
In navigation, bearings are measured relative to north. By convention, viewed from above, bearing angle are positive clockwise, so a bearing of 45° corresponds to a north-east orientation. Negative bearings are not used in navigation, so a north-west orientation corresponds to a bearing of 315°.
Alternative ways of measuring the size of an angle
There are several alternatives to measuring the size of an angle by the corresponding angle of rotation. The grade of a slope, or gradient, is equal to the tangent of the angle, or sometimes (rarely) the sine. Gradients are often expressed as a percentage. For very small values (less than 5%), the grade of a slope is approximately the measure of an angle in radians.
In rational geometry, the spread between two lines is defined at the square of sine of the angle between the lines. Since the sine of an angle and the sine of its supplementary angle are the same, any angle of rotation that maps one of the lines into the other leads to the same value of the spread between the lines.
Astronomical approximations
Astronomers measure angular separation of objects in degrees from their point of observation.
- 0.5° is approximately the width of the sun or moon.
- 1° is approximately the width of a little finger at arm's length.
- 10° is approximately the width of a closed fist at arm's length.
- 20° is approximately the width of a handspan at arm's length.
These measurements clearly depend on the individual subject, and the above should be treated as rough rule of thumb approximations only.
Measurements that are not angular units
Not all angle measurements are angular units, for an angular measurement, it is definitional that the angle addition postulate holds.
Some angle measurements where the angle addition postulate does not hold include:
Notes
- This approach requires however an additional proof that the measure of the angle does not change with changing radius r, in addition to the issue of "measurement units chosen." A smoother approach is to measure the angle by the length of the corresponding unit circle arc. Here "unit" can be chosen to be dimensionless in the sense that it is the real number 1 associated with the unit segment on the real line. See Radoslav M. Dimitrić for instance.[3]
References
- "List of Geometry and Trigonometry Symbols". Math Vault. 2020-04-17. Retrieved 2020-08-31.
- "angular unit". TheFreeDictionary.com. Retrieved 2020-08-31.
- Dimitrić, Radoslav M. (2012). "On Angles and Angle Measurements" (PDF). The Teaching of Mathematics. XV (2): 133–140. Archived (PDF) from the original on 2019-01-17. Retrieved 2019-08-06.
- Weisstein, Eric W. "Radian". mathworld.wolfram.com. Retrieved 2020-08-31.
- Bonin, Walter (2016-01-11). "RE: WP-32S in 2016?". HP Museum. Archived from the original on 2019-08-06. Retrieved 2019-08-05.
- Bonin, Walter (2019) [2015]. WP 43S Owner's Manual (PDF). 0.12 (draft ed.). pp. 72, 118–119, 311. ISBN 978-1-72950098-9. ISBN 1-72950098-6. Retrieved 2019-08-05. (314 pages)
- Bonin, Walter (2019) [2015]. WP 43S Reference Manual (PDF). 0.12 (draft ed.). pp. iii, 54, 97, 128, 144, 193, 195. ISBN 978-1-72950106-1. ISBN 1-72950106-0. Retrieved 2019-08-05. (271 pages)
- Jeans, James Hopwood (1947). The Growth of Physical Science. CUP Archive. p. 7.
- Murnaghan, Francis Dominic (1946). Analytic Geometry. p. 2.
- "ooPIC Programmer's Guide - Chapter 15: URCP". ooPIC Manual & Technical Specifications - ooPIC Compiler Ver 6.0. Savage Innovations, LLC. 2007 [1997]. Archived from the original on 2008-06-28. Retrieved 2019-08-05.
- Hargreaves, Shawn. "Angles, integers, and modulo arithmetic". blogs.msdn.com. Archived from the original on 2019-06-30. Retrieved 2019-08-05.
