Cone (topology)

Here we often use geometric cone (defined in the introduction) instead of the topological one. The considered spaces are compact, so we get the same result up to homeomorphism.

• The cone over a point p of the real line is the interval ${\displaystyle \{p\}\times [0,1]}$.
• The cone over two points {0, 1} is a "V" shape with endpoints at {0} and {1}.
• The cone over a closed interval I of the real line is a filled-in triangle (with one of the edges being I), otherwise known as a 2-simplex (see the final example).
• The cone over a polygon P is a pyramid with base P.
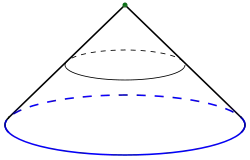
• The cone over a disk is the solid cone of classical geometry (hence the concept's name).
• The cone over a circle given by

${\displaystyle \{(x,y,z)\in \mathbb {R} ^{3}\mid x^{2}+y^{2}=1{\mbox{ and }}z=0\}}$

is the curved surface of the solid cone:

${\displaystyle \{(x,y,z)\in \mathbb {R} ^{3}\mid x^{2}+y^{2}=(z-1)^{2}{\mbox{ and }}0\leq z\leq 1\}.}$

This in turn is homeomorphic to the closed disc.

• In general, the cone over an n-sphere is homeomorphic to the closed (n + 1)-ball.
• The cone over an n-simplex is an (n + 1)-simplex.

All cones are path-connected since every point can be connected to the vertex point. Furthermore, every cone is contractible to the vertex point by the homotopy

${\displaystyle h_{t}(x,s)=(x,(1-t)s)}$.

The cone is used in algebraic topology precisely because it embeds a space as a subspace of a contractible space.

When X is compact and Hausdorff (essentially, when X can be embedded in Euclidean space), then the cone ${\displaystyle CX}$ can be visualized as the collection of lines joining every point of X to a single point. However, this picture fails when X is not compact or not Hausdorff, as generally the quotient topology on ${\displaystyle CX}$ will be finer than the set of lines joining X to a point.

The map ${\displaystyle X\mapsto CX}$ induces a functor ${\displaystyle C\colon \mathbf {Top} \to \mathbf {Top} }$ on the category of topological spaces Top. If ${\displaystyle f\colon X\to Y}$ is a continuous map, then ${\displaystyle Cf\colon CX\to CY}$ is defined by

${\displaystyle (Cf)([x,t])=[f(x),t]}$,

where square brackets denote equivalence classes.

If ${\displaystyle (X,x_{0})}$ is a pointed space, there is a related construction, the reduced cone, given by

${\displaystyle (X\times [0,1])/(X\times \left\{0\right\}\cup \left\{x_{0}\right\}\times [0,1])}$

where we take the basepoint of the reduced cone to be the equivalence class of ${\displaystyle (x_{0},0)}$. With this definition, the natural inclusion ${\displaystyle x\mapsto (x,1)}$ becomes a based map. This construction also gives a functor, from the category of pointed spaces to itself.

• Cone (disambiguation)
• Suspension (topology)
• Desuspension
• Mapping cone (topology)
• Join (topology)

• Allen Hatcher, Algebraic topology. Cambridge University Press, Cambridge, 2002. xii+544 pp. ISBN 0-521-79160-X and ISBN 0-521-79540-0
• "Cone". PlanetMath.