Doubly triangular number

The doubly triangular numbers form the sequence[1]

0, 1, 6, 21, 55, 120, 231, 406, 666, 1035, 1540, 2211, ...

The ${\displaystyle n}$th doubly triangular number has the quartic formula[2]

$${\displaystyle T_{T_{n}}={\frac {n(n+1)(n^{2}+n+2)}{8}}.}$$

The sums of row sums of Floyd's triangle give the doubly triangular numbers. Another way of expressing this fact is that the sum of all of the numbers in the first ${\displaystyle n}$ rows of Floyd's triangle is the ${\displaystyle n}$th doubly triangular number.[1][2]

Doubly triangular numbers arise naturally as numbers of unordered pairs of unordered pairs of objects, including pairs where both objects are the same:

• An example from mathematical chemistry is given by the numbers of overlap integrals between Slater-type orbitals.[3]
• Another example of this phenomenon from combinatorics is that the doubly-triangular numbers count the number of two-edge undirected multigraphs on ${\displaystyle n}$ labeled vertices. In this setting, an edge is an unordered pair of vertices, and a two-edge graph is an unordered pair of edges. The number of possible edges is a triangular number, and the number of pairs of edges (allowing both edges to connect the same two vertices) is a doubly triangular number.[4]
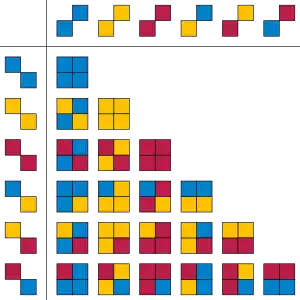
• In the same way, the doubly triangular numbers also count the number of distinct ways of coloring the four corners or the four edges of a square with ${\displaystyle n}$ colors, allowing some colors to be unused and counting two colorings as being the same when they differ from each other only by rotation or reflection of the square. The number of choices of colors for any two opposite features of the square is a triangular number, and a coloring of the whole square combines two of these colorings of pairs of opposite features.[1]

When pairs with both objects the same are excluded, a different sequence arises, the tritriangular numbers ${\textstyle {\binom {\binom {n}{2}}{2}}=3,15,45,105,\dots }$[5]

Some numerologists and biblical studies scholars consider it significant that 666, the number of the beast, is a doubly triangular number.[6][7]