Truncated cuboctahedral prism

In geometry, a truncated cuboctahedral prism or great rhombicuboctahedral prism is a convex uniform polychoron (four-dimensional polytope).

Truncated cuboctahedral prism

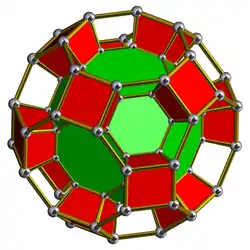Schlegel diagram
TypePrismatic uniform polychoron
Uniform index55
Schläfli symbolt0,1,2,3{4,3,2} or tr{4,3}×{}
Coxeter-Dynkin
Cells28 total:
2 4.6.8
12 4.4.4
8 4.4.6
6 4.4.8
Faces124 total:
96 {4}
16 {6}
12 {8}
Edges192
Vertices96
Vertex figure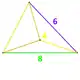
Irregular tetrahedron
Symmetry group[4,3,2], order 96
Propertiesconvex

It is one of 18 convex uniform polyhedral prisms created by using uniform prisms to connect pairs of Platonic solids or Archimedean solids in parallel hyperplanes.


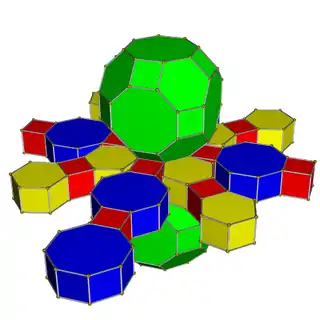
Net

Alternative names
  • Truncated-cuboctahedral dyadic prism (Norman W. Johnson)
  • Gircope (Jonathan Bowers: for great rhombicuboctahedral prism/hyperprism)
  • Great rhombicuboctahedral prism/hyperprism

A full snub cubic antiprism or omnisnub cubic antiprism can be defined as an alternation of an truncated cuboctahedral prism, represented by ht0,1,2,3{4,3,2}, or , although it cannot be constructed as a uniform polychoron. It has 76 cells: 2 snub cubes connected by 12 tetrahedrons, 6 square antiprisms, and 8 octahedrons, with 48 tetrahedrons in the alternated gaps. There are 48 vertices, 192 edges, and 220 faces (12 squares, and 16+192 triangles). It has [4,3,2]+ symmetry, order 48.

A construction exists with two uniform snub cubes in snub positions with two edge lengths in a ratio of around 1 : 1.138.


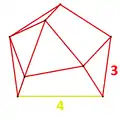
Vertex figure for the omnisnub cubic antiprism

Also related is the bialternatosnub octahedral hosochoron, constructed by removing alternating long rectangles from the octagons, but is also not uniform. It has 40 cells: 2 rhombicuboctahedra (with Th symmetry), 6 rectangular trapezoprisms (topologically equivalent to a cube but with D2d symmetry), 8 octahedra (as triangular antiprisms), 24 triangular prisms (as Cs-symmetry wedges) filling the gaps, and 48 vertices. It has [4,(3,2)+] symmetry, order 48. Its vertex figure is a chiral hexahedron topologically identical to the tetragonal antiwedge.


Vertex figure for the bialternatosnub octahedral hosochoron

  • 6. Convex uniform prismatic polychora - Model 55, George Olshevsky.
  • Klitzing, Richard. "4D uniform polytopes (polychora) x3x4x x - gircope".


This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.