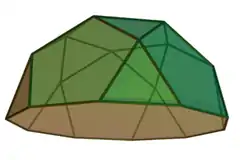
Pentagonal orthobirotunda
In geometry, the pentagonal orthobirotunda is one of the Johnson solids (J34). It can be constructed by joining two pentagonal rotundae (J6) along their decagonal faces, matching like faces.
| Pentagonal orthobirotunda | |
|---|---|
 | |
| Type | Johnson J33 - J34 - J35 |
| Faces | 2x10 triangles 2+10 pentagons |
| Edges | 60 |
| Vertices | 30 |
| Vertex configuration | 10(32.52) 2.10(3.5.3.5) |
| Symmetry group | D5h |
| Dual polyhedron | Trapezo-rhombic triacontahedron |
| Properties | convex |
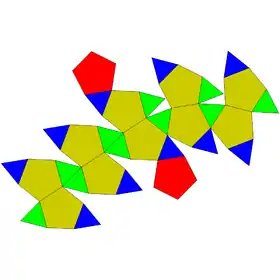
| Net | |
 | |
A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[1]
Related polyhedra
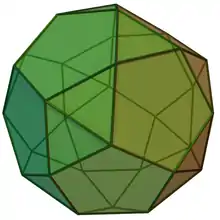
The pentagonal orthobirotunda is also related to an Archimedean solid, the icosidodecahedron, which can also be called a pentagonal gyrobirotunda, similarly created by two pentagonal rotunda but with a 36-degree rotation.
 (Dissection) |
|
External links
- Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.