List of unsolved problems in physics

The following is a list of notable unsolved problems grouped into broad areas of physics.[1]

This is a dynamic list and may never be able to satisfy particular standards for completeness. You can help by adding missing items with reliable sources.

Some of the major unsolved problems in physics are theoretical, meaning that existing theories seem incapable of explaining a certain observed phenomenon or experimental result. The others are experimental, meaning that there is a difficulty in creating an experiment to test a proposed theory or investigate a phenomenon in greater detail.

There are still some questions beyond the Standard Model of physics, such as the strong CP problem, neutrino mass, matter–antimatter asymmetry, and the nature of dark matter and dark energy.[2][3] Another problem lies within the mathematical framework of the Standard Model itself—the Standard Model is inconsistent with that of general relativity, to the point that one or both theories break down under certain conditions (for example within known spacetime singularities like the Big Bang and the centres of black holes beyond the event horizon).

General physics/quantum physics
  • Theory of everything: Is there a theory which explains the values of all fundamental physical constants, i.e., of all coupling constants, all elementary particle masses and all mixing angles of elementary particles?[4] Is there a theory which explains why the gauge groups of the standard model are as they are, and why observed spacetime has 3 spatial dimensions and 1 temporal dimension? Are "fundamental physical constants" really fundamental or do they vary over time? Are any of the fundamental particles in the standard model of particle physics actually composite particles too tightly bound to observe as such at current experimental energies? Are there elementary particles that have not yet been observed, and, if so, which ones are they and what are their properties? Are there unobserved fundamental forces?
  • Arrow of time (e.g. entropy's arrow of time): Why does time have a direction? Why did the universe have such low entropy in the past, and time correlates with the universal (but not local) increase in entropy, from the past and to the future, according to the second law of thermodynamics?[4] Why are CP violations observed in certain weak force decays, but not elsewhere? Are CP violations somehow a product of the second law of thermodynamics, or are they a separate arrow of time? Are there exceptions to the principle of causality? Is there a single possible past? Is the present moment physically distinct from the past and future, or is it merely an emergent property of consciousness? What links the quantum arrow of time to the thermodynamic arrow?
  • Interpretation of quantum mechanics: How does the quantum description of reality, which includes elements such as the superposition of states and wavefunction collapse or quantum decoherence, give rise to the reality we perceive?[4] Another way of stating this question regards the measurement problem: What constitutes a "measurement" which apparently causes the wave function to collapse into a definite state? Unlike classical physical processes, some quantum mechanical processes (such as quantum teleportation arising from quantum entanglement) cannot be simultaneously "local", "causal", and "real", but it is not obvious which of these properties must be sacrificed,[5] or if an attempt to describe quantum mechanical processes in these senses is a category error such that a proper understanding of quantum mechanics would render the question meaningless. Can a multiverse resolve it?
  • Yang–Mills theory: Given an arbitrary compact gauge group, does a non-trivial quantum Yang–Mills theory with a finite mass gap exist? (This problem is also listed as one of the Millennium Prize Problems in mathematics.)[6]
  • Color confinement: The quantum chromodynamics (QCD) color confinement conjecture is that color charged particles (such as quarks and gluons) cannot be separated from their parent hadron without producing new hadrons.[7] Is it possible to provide an analytic proof of color confinement in any non-abelian gauge theory?
  • Dimensionless physical constant: At the present time, the values of the dimensionless physical constants cannot be calculated; they are determined only by physical measurement.[8][9] What is the minimum number of dimensionless physical constants from which all other dimensionless physical constants can be derived? Are dimensional physical constants necessary at all?
  • Fine-tuned universe: The values of the fundamental physical constants are in a narrow range necessary to support carbon-based life.[10][11][12] Is this because there exist other universes with different constants, or are our universe's constants the result of chance, or some other factor or process? In particular, Tegmark's mathematical multiverse hypothesis of abstract mathematical parallel universe formalized models, and the landscape multiverse hypothesis of spacetime regions having different formalized sets of laws and physical constants from that of the surrounding space—require formalization.
  • Quantum field theory: Is it possible to construct, in the mathematically rigorous framework of algebraic QFT, a theory in 4-dimensional spacetime that includes interactions and does not resort to perturbative methods?[13][14]
  • Locality: Are there non-local phenomena in quantum physics?[15][16] If they exist, are non-local phenomena limited to the entanglement revealed in the violations of the Bell inequalities, or can information and conserved quantities also move in a non-local way? Under what circumstances are non-local phenomena observed? What does the existence or absence of non-local phenomena imply about the fundamental structure of spacetime? How does this elucidate the proper interpretation of the fundamental nature of quantum physics?

Cosmology and general relativity
Estimated distribution of dark matter and dark energy in the universe
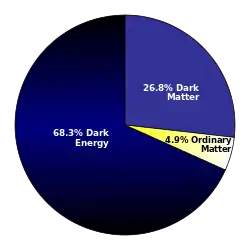
  • Dark matter: What is the identity of dark matter?[19] Is it a particle? Is it the lightest superpartner (LSP)? Or, do the phenomena attributed to dark matter point not to some form of matter but actually to an extension of gravity?
  • Dark energy: What is the cause of the observed accelerated expansion (de Sitter phase) of the universe? Why is the energy density of the dark energy component of the same magnitude as the density of matter at present when the two evolve quite differently over time; could it be simply that we are observing at exactly the right time? Is dark energy a pure cosmological constant or are models of quintessence such as phantom energy applicable?
  • Dark flow: Is a non-spherically symmetric gravitational pull from outside the observable universe responsible for some of the observed motion of large objects such as galactic clusters in the universe?
  • Axis of evil: Some large features of the microwave sky at distances of over 13 billion light years appear to be aligned with both the motion and orientation of the solar system. Is this due to systematic errors in processing, contamination of results by local effects, or an unexplained violation of the Copernican principle?
  • Shape of the universe: What is the 3-manifold of comoving space, i.e. of a comoving spatial section of the universe, informally called the "shape" of the universe? Neither the curvature nor the topology is presently known, though the curvature is known to be "close" to zero on observable scales. The cosmic inflation hypothesis suggests that the shape of the universe may be unmeasurable, but, since 2003, Jean-Pierre Luminet, et al., and other groups have suggested that the shape of the universe may be the Poincaré dodecahedral space. Is the shape unmeasurable; the Poincaré space; or another 3-manifold?
  • The largest structures in the universe are larger than expected. Current cosmological models say there should be very little structure on scales larger than a few hundred million light years across, due to the expansion of the universe trumping the effect of gravity.[23] But the Sloan Great Wall is 1.38 billion light-years in length. And the largest structure currently known, the Hercules–Corona Borealis Great Wall, is up to 10 billion light-years in length. Are these actual structures or random density fluctuations? If they are real structures, they contradict the 'End of Greatness' hypothesis which asserts that at a scale of 300 million light-years structures seen in smaller surveys are randomized to the extent that the smooth distribution of the universe is visually apparent.
  • Extra dimensions: Does nature have more than four spacetime dimensions? If so, what is their size? Are dimensions a fundamental property of the universe or an emergent result of other physical laws? Can we experimentally observe evidence of higher spatial dimensions?

Quantum gravity

High-energy physics/particle physics
See also: Beyond the Standard Model
  • Hierarchy problem: Why is gravity such a weak force? It becomes strong for particles only at the Planck scale, around 1019 GeV, much above the electroweak scale (100 GeV, the energy scale dominating physics at low energies). Why are these scales so different from each other? What prevents quantities at the electroweak scale, such as the Higgs boson mass, from getting quantum corrections on the order of the Planck scale? Is the solution supersymmetry, extra dimensions, or just anthropic fine-tuning?
  • Planck particle: The Planck mass plays an important role in parts of mathematical physics. A series of researchers have suggested the existence of a fundamental particle with mass equal to or close to that of the Planck mass. The Planck mass is however enormous compared to any detected particle. It is still an unsolved problem if there exist or even have existed a particle with close to the Planck mass. This is indirectly related to the hierarchy problem.
  • Magnetic monopoles: Did particles that carry "magnetic charge" exist in some past, higher-energy epoch? If so, do any remain today? (Paul Dirac showed the existence of some types of magnetic monopoles would explain charge quantization.)[27]
  • Neutron lifetime puzzle: While the neutron lifetime has been studied for decades, there currently exists a lack of consilience on its exact value, due to different results from two experimental methods ("bottle" versus "beam").[28]
  • Proton decay and spin crisis: Is the proton fundamentally stable? Or does it decay with a finite lifetime as predicted by some extensions to the standard model?[29] How do the quarks and gluons carry the spin of protons?[30]
  • Supersymmetry: Is spacetime supersymmetry realized at TeV scale? If so, what is the mechanism of supersymmetry breaking? Does supersymmetry stabilize the electroweak scale, preventing high quantum corrections? Does the lightest supersymmetric particle (LSP) comprise dark matter?
  • Generations of matter: Why are there three generations of quarks and leptons? Is there a theory that can explain the masses of particular quarks and leptons in particular generations from first principles (a theory of Yukawa couplings)?[31]
  • Neutrino mass: What is the mass of neutrinos, whether they follow Dirac or Majorana statistics? Is the mass hierarchy normal or inverted? Is the CP violating phase equal to 0?[32][33]
  • Strong CP problem and axions: Why is the strong nuclear interaction invariant to parity and charge conjugation? Is Peccei–Quinn theory the solution to this problem? Could axions be the main component of dark matter?
  • Anomalous magnetic dipole moment: Why is the experimentally measured value of the muon's anomalous magnetic dipole moment ("muon g−2") significantly different from the theoretically predicted value of that physical constant?[34]
  • Proton radius puzzle: What is the electric charge radius of the proton? How does it differ from gluonic charge?
  • Pentaquarks and other exotic hadrons: What combinations of quarks are possible? Why were pentaquarks so difficult to discover?[35] Are they a tightly-bound system of five elementary particles, or a more weakly-bound pairing of a baryon and a meson?[36]
  • Mu problem: problem of supersymmetric theories, concerned with understanding the parameters of the theory.
  • Koide formula: An aspect of the problem of particle generations. The sum of the masses of the three charged leptons, divided by the square of the sum of the roots of these masses, to within one standard deviation of observations, is . It is unknown how such a simple value comes about, and why it is the exact arithmetic average of the possible extreme values of ​1⁄3 (equal masses) and 1 (one mass dominates).

Astronomy and astrophysics
Main article: List of unsolved problems in astronomy
  • Solar cycle: How does the Sun generate its periodically reversing large-scale magnetic field? How do other solar-like stars generate their magnetic fields, and what are the similarities and differences between stellar activity cycles and that of the Sun?[37] What caused the Maunder Minimum and other grand minima, and how does the solar cycle recover from a minima state?
  • Coronal heating problem: Why is the Sun's corona (atmosphere layer) so much hotter than the Sun's surface? Why is the magnetic reconnection effect many orders of magnitude faster than predicted by standard models?
  • Astrophysical jet: Why do only certain accretion discs surrounding certain astronomical objects emit relativistic jets along their polar axes? Why are there quasi-periodic oscillations in many accretion discs?[38] Why does the period of these oscillations scale as the inverse of the mass of the central object?[39] Why are there sometimes overtones, and why do these appear at different frequency ratios in different objects?[40]
  • Diffuse interstellar bands: What is responsible for the numerous interstellar absorption lines detected in astronomical spectra? Are they molecular in origin, and if so which molecules are responsible for them? How do they form?
  • Supermassive black holes: What is the origin of the M-sigma relation between supermassive black hole mass and galaxy velocity dispersion?[41] How did the most distant quasars grow their supermassive black holes up to 1010 solar masses so early in the history of the universe?
Rotation curve of a typical spiral galaxy: predicted (A) and observed (B). Can the discrepancy between the curves be attributed to dark matter?
  • Kuiper cliff: Why does the number of objects in the Solar System's Kuiper belt fall off rapidly and unexpectedly beyond a radius of 50 astronomical units?
  • Flyby anomaly: Why is the observed energy of satellites flying by planetary bodies sometimes different by a minute amount from the value predicted by theory?
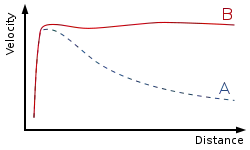
  • Galaxy rotation problem: Is dark matter responsible for differences in observed and theoretical speed of stars revolving around the centre of galaxies, or is it something else?
  • Supernovae: What is the exact mechanism by which an implosion of a dying star becomes an explosion?
  • p-nuclei: What astrophysical process is responsible for the nucleogenesis of these rare isotopes?
  • Ultra-high-energy cosmic ray:[19] Why is it that some cosmic rays appear to possess energies that are impossibly high, given that there are no sufficiently energetic cosmic ray sources near the Earth? Why is it that (apparently) some cosmic rays emitted by distant sources have energies above the Greisen–Zatsepin–Kuzmin limit?[4][19]
  • Rotation rate of Saturn: Why does the magnetosphere of Saturn exhibit a (slowly changing) periodicity close to that at which the planet's clouds rotate? What is the true rotation rate of Saturn's deep interior?[42]
  • Origin of magnetar magnetic field: What is the origin of magnetar magnetic field?
  • Large-scale anisotropy: Is the universe at very large scales anisotropic, making the cosmological principle an invalid assumption? The number count and intensity dipole anisotropy in radio, NRAO VLA Sky Survey (NVSS) catalogue[43] is inconsistent with the local motion as derived from cosmic microwave background[44][45] and indicate an intrinsic dipole anisotropy. The same NVSS radio data also shows an intrinsic dipole in polarization density and degree of polarization[46] in the same direction as in number count and intensity. There are several other observations revealing large-scale anisotropy. The optical polarization from quasars shows polarization alignment over a very large scale of Gpc.[47][48][49] The cosmic-microwave-background data shows several features of anisotropy,[50][51][52][53] which are not consistent with the Big Bang model.
  • Age–metallicity relation in the Galactic disk: Is there a universal age–metallicity relation (AMR) in the Galactic disk (both "thin" and "thick" parts of the disk)? Although in the local (primarily thin) disk of the Milky Way there is no evidence of a strong AMR,[54] a sample of 229 nearby "thick" disk stars has been used to investigate the existence of an age–metallicity relation in the Galactic thick disk, and indicate that there is an age–metallicity relation present in the thick disk.[55][56] Stellar ages from asteroseismology confirm the lack of any strong age–metallicity relation in the Galactic disc.[57]
  • The lithium problem: Why is there a discrepancy between the amount of lithium-7 predicted to be produced in Big Bang nucleosynthesis and the amount observed in very old stars?[58]
  • Ultraluminous X-ray sources (ULXs): What powers X-ray sources that are not associated with active galactic nuclei but exceed the Eddington limit of a neutron star or stellar black hole? Are they due to intermediate mass black holes? Some ULXs are periodic, suggesting non-isotropic emission from a neutron star. Does this apply to all ULXs? How could such a system form and remain stable?
  • Fast radio bursts (FRBs): What causes these transient radio pulses from distant galaxies, lasting only a few milliseconds each? Why do some FRBs repeat at unpredictable intervals, but most do not? Dozens of models have been proposed, but none have been widely accepted.[59]

Nuclear physics
The "island of stability" in the proton vs. neutron number plot for heavy nuclei
  • Quantum chromodynamics: What are the phases of strongly interacting matter, and what roles do they play in the evolution of cosmos? What is the detailed partonic structure of the nucleons? What does QCD predict for the properties of strongly interacting matter? What determines the key features of QCD, and what is their relation to the nature of gravity and spacetime? Do glueballs exist? Do gluons acquire mass dynamically despite having a zero rest mass, within hadrons? Does QCD truly lack CP violations?
  • Quark-gluon plasma: Where is the onset of deconfinement: 1) as a function of temperature and chemical potentials? 2) as a function of relativistic heavy-ion collision energy and system size? What is the mechanism of energy and baryon-number stopping leading to creation of quark-gluon plasma in relativistic heavy-ion collisions? Why is sudden hadronization and the statistical-hadronization model a near-to-perfect description of hadron production from quark-gluon plasma? Is quark flavor conserved in quark-gluon plasma? Are strangeness and charm in chemical equilibrium in quark-gluon plasma? Does strangeness in quark-gluon plasma flow at the same speed as up and down quark flavours? Why does deconfined matter show ideal flow?
  • Strangelets: Does strange quark matter (Strangelet) exist as stable state?
  • Specific models of quark-gluon plasma formation: Do gluons saturate when their occupation number is large? Do gluons form a dense system called Colour Glass Condensate? What are the signatures and evidences for the Balitsky–Fadin–Kuarev–Lipatov, Balitsky–Kovchegov, Catani–Ciafaloni–Fiorani–Marchesini evolution equations?
  • Nuclei and nuclear astrophysics: Why is there a lack of convergence in estimates of the mean lifetime of a free neutron based on two separate—and increasingly precise—experimental methods? What is the nature of the nuclear force that binds protons and neutrons into stable nuclei and rare isotopes? What is the nature of exotic excitations in nuclei at the frontiers of stability and their role in stellar processes? What is the nature of neutron stars and dense nuclear matter? What is the origin of the elements in the cosmos? What are the nuclear reactions that drive stars and stellar explosions? What is the heaviest possible chemical element?

Atomic, molecular and optical physics

Fluid dynamics
  • Under what conditions do smooth solutions exist for the Navier–Stokes equations, which are the equations that describe the flow of an incompressible fluid? This problem is also listed as one of the Millennium Prize Problems in mathematics.
  • Turbulent flow: Is it possible to make a theoretical model to describe the statistics of a turbulent flow (in particular, its internal structures)?[4]
  • Upstream contamination: When pouring water from a higher container to a lower one, particles floating in the latter can climb upstream into the upper container. A definitive explanation for this phenomenon is still lacking.

Condensed matter physics
A sample of a cuprate superconductor (specifically BSCCO). The mechanism for superconductivity of these materials is unknown.
Magnetoresistance in a fractional quantum Hall state.

Plasma physics
  • Plasma physics and fusion power: Fusion energy may potentially provide power from abundant resource (e.g. hydrogen) without the type of radioactive waste that fission energy currently produces. However, can ionized gases (plasma) be confined long enough and at a high enough temperature to create fusion power? What is the physical origin of H-mode?[78]
  • The injection problem: Fermi acceleration is thought to be the primary mechanism that accelerates astrophysical particles to high energy. However, it is unclear what mechanism causes those particles to initially have energies high enough for Fermi acceleration to work on them.[79]
  • Alfvénic turbulence: In the solar wind and the turbulence in solar flares, coronal mass ejections, and magnetospheric substorms are major unsolved problems in space plasma physics.[80]

Biophysics

See also

References
  1. Ginzburg, Vitaly L. (2001). The physics of a lifetime : reflections on the problems and personalities of 20th century physics. Berlin: Springer. pp. 3–200. ISBN 978-3-540-67534-1.
  2. Hammond, Richard (1 May 2008). "The Unknown Universe: The Origin of the Universe, Quantum Gravity, Wormholes, and Other Things Science Still Can't Explain". Proceedings of the Royal Society of London, Series A. 456 (1999): 1685.
  3. Womersley, J. (February 2005). "Beyond the Standard Model" (PDF). Symmetry Magazine. Archived from the original (PDF) on 17 October 2007. Retrieved 23 November 2010.
  4. Baez, John C. (March 2006). "Open Questions in Physics". Usenet Physics FAQ. University of California, Riverside: Department of Mathematics. Retrieved 7 March 2011.
  5. Cabello, Adán (2017). "Interpretations of quantum theory: A map of madness". In Lombardi, Olimpia; Fortin, Sebastian; Holik, Federico; López, Cristian (eds.). What is Quantum Information?. Cambridge University Press. pp. 138–143. arXiv:1509.04711. Bibcode:2015arXiv150904711C. doi:10.1017/9781316494233.009. ISBN 9781107142114. S2CID 118419619.
  6. "Yang–Mills and Mass Gap". Clay Mathematics Institute. Retrieved 31 January 2018.
  7. Wu, T.-Y.; Pauchy Hwang, W.-Y. (1991). Relativistic quantum mechanics and quantum fields. World Scientific. p. 321. ISBN 978-981-02-0608-6.
  8. "Alcohol constrains physical constant in the early universe". Phys Org. 13 December 2012. Retrieved 25 March 2015.
  9. Bagdonaite, J.; Jansen, P.; Henkel, C.; Bethlem, H. L.; Menten, K. M.; Ubachs, W. (13 December 2012). "A Stringent Limit on a Drifting Proton-to-Electron Mass Ratio from Alcohol in the Early Universe". Science. 339 (6115): 46–48. Bibcode:2013Sci...339...46B. doi:10.1126/science.1224898. hdl:1871/39591. PMID 23239626. S2CID 716087.
  10. Rees, Martin (3 May 2001). Just Six Numbers: The Deep Forces That Shape The Universe. New York, NY: Basic Books; First American edition. pp. 4.
  11. Gribbin. J and Rees. M, Cosmic Coincidences: Dark Matter, Mankind, and Anthropic Cosmology p. 7, 269, 1989, ISBN 0-553-34740-3
  12. Davis, Paul (2007). Cosmic Jackpot: Why Our Universe Is Just Right for Life. New York, NY: Orion Publications. pp. 2. ISBN 978-0618592265.
  13. Rejzner, Kasia (2016). Perturbative Algebraic Quantum Field Theory. Mathematical Physics Studies. Springer. arXiv:1208.1428. doi:10.1007/978-3-319-25901-7. ISBN 978-3-319-25899-7.
  14. Fredenhagen, Klaus; Rejzner, Katarzyna (26 March 2015). "Perturbative Construction of Models of Algebraic Quantum Field Theory". arXiv:1503.07814 [math-ph].
  15. Wiseman, Howard (2014). "The Two Bell's Theorems of John Bell". Journal of Physics A: Mathematical and Theoretical. 47 (42): 424001. arXiv:1402.0351. Bibcode:2014JPhA...47P4001W. doi:10.1088/1751-8113/47/42/424001. ISSN 1751-8121. S2CID 119234957.
  16. Fuchs, Christopher A.; Mermin, N. David; Schack, Rüdiger (2014). "An introduction to QBism with an application to the locality of quantum mechanics". American Journal of Physics. 82 (8): 749. arXiv:1311.5253. Bibcode:2014AmJPh..82..749F. doi:10.1119/1.4874855. S2CID 56387090.
  17. Isham, C. J. (1993). "Canonical Quantum Gravity and the Problem of Time". Integrable Systems, Quantum Groups, and Quantum Field Theories. NATO ASI Series. Springer, Dordrecht. pp. 157–287. arXiv:gr-qc/9210011. doi:10.1007/978-94-011-1980-1_6. ISBN 9789401048743. S2CID 116947742.
  18. Podolsky, Dmitry. "Top ten open problems in physics". NEQNET. Archived from the original on 22 October 2012. Retrieved 24 January 2013.
  19. Brooks, Michael (19 March 2005). "13 Things That Do Not Make Sense". New Scientist. Issue 2491. Retrieved 7 March 2011.
  21. Steinhardt, P. & Turok, N. (2006). "Why the Cosmological constant is so small and positive". Science. 312 (5777): 1180–1183. arXiv:astro-ph/0605173. Bibcode:2006Sci...312.1180S. doi:10.1126/science.1126231. PMID 16675662. S2CID 14178620.
  22. Wang, Qingdi; Zhu, Zhen; Unruh, William G. (11 May 2017). "How the huge energy of quantum vacuum gravitates to drive the slow accelerating expansion of the Universe". Physical Review D. 95 (10): 103504. arXiv:1703.00543. Bibcode:2017PhRvD..95j3504W. doi:10.1103/PhysRevD.95.103504. S2CID 119076077. This problem is widely regarded as one of the major obstacles to further progress in fundamental physics [...] Its importance has been emphasized by various authors from different aspects. For example, it has been described as a “veritable crisis” [...] and even “the mother of all physics problems” [...] While it might be possible that people working on a particular problem tend to emphasize or even exaggerate its importance, those authors all agree that this is a problem that needs to be solved, although there is little agreement on what is the right direction to find the solution.
  23. Stephen Battersby (21 June 2011). "Largest cosmic structures 'too big' for theories". New Scientist. Retrieved 5 July 2019
  24. Alan Sokal (22 July 1996). "Don't Pull the String Yet on Superstring Theory". New York Times.
  25. Peres, Asher; Terno, Daniel R. (2004). "Quantum information and relativity theory". Reviews of Modern Physics. 76 (1): 93–123. arXiv:quant-ph/0212023. Bibcode:2004RvMP...76...93P. doi:10.1103/revmodphys.76.93. S2CID 7481797.
  26. Joshi, Pankaj S. (January 2009). "Do Naked Singularities Break the Rules of Physics?". Scientific American. Archived from the original on 25 May 2012.
  27. Dirac, Paul, "Quantised Singularities in the Electromagnetic Field". Proceedings of the Royal Society A 133, 60 (1931).
  28. Wolchover, Natalie (13 February 2018). "Neutron Lifetime Puzzle Deepens, but No Dark Matter Seen". Quanta Magazine. Retrieved 31 July 2018. When physicists strip neutrons from atomic nuclei, put them in a bottle, then count how many remain there after some time, they infer that neutrons radioactively decay in 14 minutes and 39 seconds, on average. But when other physicists generate beams of neutrons and tally the emerging protons—the particles that free neutrons decay into—they peg the average neutron lifetime at around 14 minutes and 48 seconds. The discrepancy between the “bottle” and “beam” measurements has persisted since both methods of gauging the neutron’s longevity began yielding results in the 1990s. At first, all the measurements were so imprecise that nobody worried. Gradually, though, both methods have improved, and still they disagree.
  29. Li, Tianjun; Dimitri V. Nanopoulos; Joel W. Walker (2011). "Elements of F-ast Proton Decay". Nuclear Physics B. 846 (1): 43–99. arXiv:1003.2570. Bibcode:2011NuPhB.846...43L. doi:10.1016/j.nuclphysb.2010.12.014. S2CID 119246624.
  30. Hansson, Johan (2010). "The "Proton Spin Crisis" – a Quantum Query" (PDF). Progress in Physics. 3. Archived from the original (PDF) on 4 May 2012. Retrieved 14 April 2012.
  31. A. Blumhofer; M. Hutter (1997). "Family Structure from Periodic Solutions of an Improved Gap Equation". Nuclear Physics. B484 (1): 80–96. Bibcode:1997NuPhB.484...80B. CiteSeerX 10.1.1.343.783. doi:10.1016/S0550-3213(96)00644-X.
  32. "India-based Neutrino Observatory (INO)". Tata Institute of Fundamental Research. Retrieved 14 April 2012.
  33. Nakamura (Particle Data Group), K; et al. (2010). "2011 Review of Particle Physics". J. Phys. G. 37 (7A): 075021. Bibcode:2010JPhG...37g5021N. doi:10.1088/0954-3899/37/7A/075021.
  34. Thomas Blum; Achim Denig; Ivan Logashenko; Eduardo de Rafael; Lee Roberts, B.; Thomas Teubner; Graziano Venanzoni (2013). "The Muon (g-2) Theory Value: Present and Future". arXiv:1311.2198 [hep-ph].
  35. H. Muir (2 July 2003). "Pentaquark discovery confounds sceptics". New Scientist. Retrieved 8 January 2010.
  36. G. Amit (14 July 2015). "Pentaquark discovery at LHC shows long-sought new form of matter". New Scientist. Retrieved 14 July 2015.
  37. Michael J. Thompson (2014). "Grand Challenges in the Physics of the Sun and Sun-like Stars". Frontiers in Astronomy and Space Sciences. 1: 1. arXiv:1406.4228. Bibcode:2014FrASS...1....1T. doi:10.3389/fspas.2014.00001. S2CID 1547625.
  38. Strohmayer, Tod E.; Mushotzky, Richard F. (20 March 2003). "Discovery of X-Ray Quasi-periodic Oscillations from an Ultraluminous X-Ray Source in M82: Evidence against Beaming". The Astrophysical Journal. 586 (1): L61–L64. arXiv:astro-ph/0303665. Bibcode:2003ApJ...586L..61S. doi:10.1086/374732. S2CID 118992703.
  39. Titarchuk, Lev; Fiorito, Ralph (10 September 2004). "Spectral Index and Quasi‐Periodic Oscillation Frequency Correlation in Black Hole Sources: Observational Evidence of Two Phases and Phase Transition in Black Holes" (PDF). The Astrophysical Journal. 612 (2): 988–999. arXiv:astro-ph/0405360. Bibcode:2004ApJ...612..988T. doi:10.1086/422573. S2CID 4689535. Archived from the original (PDF) on 3 February 2014. Retrieved 25 January 2013.
  40. Shoji Kato (2012). "An Attempt to Describe Frequency Correlations among kHz QPOs and HBOs by Two-Armed Nearly Vertical Oscillations". Publications of the Astronomical Society of Japan. 64 (3): 62. arXiv:1202.0121. Bibcode:2012PASJ...64...62K. doi:10.1093/pasj/64.3.62. S2CID 118498018.
  41. Ferrarese, Laura; Merritt, David (2000). "A Fundamental Relation between Supermassive Black Holes and their Host Galaxies". The Astrophysical Journal. 539 (1): L9–L12. arXiv:astro-ph/0006053. Bibcode:2000ApJ...539L...9F. doi:10.1086/312838. S2CID 6508110.
  42. "Scientists Find That Saturn's Rotation Period is a Puzzle". NASA. 28 June 2004. Retrieved 22 March 2007.
  43. Condon, J. J.; Cotton, W. D.; Greisen, E. W.; Yin, Q. F.; Perley, R. A.; Taylor, G. B.; Broderick, J. J. (1998). "The NRAO VLA Sky Survey". The Astronomical Journal. 115 (5): 1693–1716. Bibcode:1998AJ....115.1693C. doi:10.1086/300337.
  44. Singal, Ashok K. (2011). "Large peculiar motion of the solar system from the dipole anisotropy in sky brightness due to distant radio sources". The Astrophysical Journal. 742 (2): L23–L27. arXiv:1110.6260. Bibcode:2011ApJ...742L..23S. doi:10.1088/2041-8205/742/2/L23. S2CID 119117071.
  45. Tiwari, Prabhakar; Kothari, Rahul; Naskar, Abhishek; Nadkarni-Ghosh, Sharvari; Jain, Pankaj (2015). "Dipole anisotropy in sky brightness and source count distribution in radio NVSS data". Astroparticle Physics. 61: 1–11. arXiv:1307.1947. Bibcode:2015APh....61....1T. doi:10.1016/j.astropartphys.2014.06.004. S2CID 119203300.
  46. Tiwari, P.; Jain, P. (2015). "Dipole anisotropy in integrated linearly polarized flux density in NVSS data". Monthly Notices of the Royal Astronomical Society. 447 (3): 2658–2670. arXiv:1308.3970. Bibcode:2015MNRAS.447.2658T. doi:10.1093/mnras/stu2535. S2CID 118610706.
  47. Hutsemekers, D. (1998). "Evidence for very large-scale coherent orientations of quasar polarization vectors". Astronomy and Astrophysics. 332: 410–428. Bibcode:1998A&A...332..410H.
  48. Hutsemékers, D.; Lamy, H. (2001). "Confirmation of the existence of coherent orientations of quasar polarization vectors on cosmological scales". Astronomy & Astrophysics. 367 (2): 381–387. arXiv:astro-ph/0012182. Bibcode:2001A&A...367..381H. doi:10.1051/0004-6361:20000443. S2CID 17157567.
  49. Jain, P.; Narain, G.; Sarala, S. (2004). "Large-scale alignment of optical polarizations from distant QSOs using coordinate-invariant statistics". Monthly Notices of the Royal Astronomical Society. 347 (2): 394–402. arXiv:astro-ph/0301530. Bibcode:2004MNRAS.347..394J. doi:10.1111/j.1365-2966.2004.07169.x. S2CID 14190653.
  50. Angelica de Oliveira-Costa; Tegmark, Max; Zaldarriaga, Matias; Hamilton, Andrew (2004). "The significance of the largest scale CMB fluctuations in WMAP". Physical Review D. 69 (6): 063516. arXiv:astro-ph/0307282. Bibcode:2004PhRvD..69f3516D. doi:10.1103/PhysRevD.69.063516. S2CID 119463060.
  51. Eriksen, H. K.; Hansen, F. K.; Banday, A. J.; Górski, K. M.; Lilje, P. B. (2004). "Asymmetries in the Cosmic Microwave Background Anisotropy Field". The Astrophysical Journal. 605 (1): 14–20. arXiv:astro-ph/0307507. Bibcode:2004ApJ...605...14E. doi:10.1086/382267.
  52. Pramoda Kumar Samal; Saha, Rajib; Jain, Pankaj; Ralston, John P. (2008). "Testing Isotropy of Cosmic Microwave Background Radiation". Monthly Notices of the Royal Astronomical Society. 385 (4): 1718–1728. arXiv:0708.2816. Bibcode:2008MNRAS.385.1718S. doi:10.1111/j.1365-2966.2008.12960.x. S2CID 988092.
  53. Pramoda Kumar Samal; Saha, Rajib; Jain, Pankaj; Ralston, John P. (2009). "Signals of Statistical Anisotropy in WMAP Foreground-Cleaned Maps". Monthly Notices of the Royal Astronomical Society. 396 (511): 511–522. arXiv:0811.1639. Bibcode:2009MNRAS.396..511S. doi:10.1111/j.1365-2966.2009.14728.x. S2CID 16250321.
  54. Casagrande, L.; Schönrich, R.; Asplund, M.; Cassisi, S.; Ramírez, I.; Meléndez, J.; Bensby, T.; Feltzing, S. (2011). "New constraints on the chemical evolution of the solar neighbourhood and Galactic disc(s)". Astronomy & Astrophysics. 530: A138. arXiv:1103.4651. Bibcode:2011A&A...530A.138C. doi:10.1051/0004-6361/201016276. S2CID 56118016.
  55. Bensby, T.; Feltzing, S.; Lundström, I. (July 2004). "A possible age–metallicity relation in the Galactic thick disk?". Astronomy and Astrophysics. 421 (3): 969–976. arXiv:astro-ph/0403591. Bibcode:2004A&A...421..969B. doi:10.1051/0004-6361:20035957. S2CID 10469794.
  56. Gilmore, G.; Asiri, H. M. (2011). "Open Issues in the Evolution of the Galactic Disks". Stellar Clusters & Associations: A RIA Workshop on Gaia. Proceedings. Granada: 280. Bibcode:2011sca..conf..280G.
  57. Casagrande, L.; Silva Aguirre, V.; Schlesinger, K. J.; Stello, D.; Huber, D.; Serenelli, A. M.; Scho Nrich, R.; Cassisi, S.; Pietrinferni, A.; Hodgkin, S.; Milone, A. P.; Feltzing, S.; Asplund, M. (2015). "Measuring the vertical age structure of the Galactic disc using asteroseismology and SAGA". Monthly Notices of the Royal Astronomical Society. 455 (1): 987–1007. arXiv:1510.01376. Bibcode:2016MNRAS.455..987C. doi:10.1093/mnras/stv2320. S2CID 119113283.
  58. Fields, Brian D. (2012). "The Primordial Lithium Problem". Annual Review of Nuclear and Particle Science. 61 (2011): 47–68. arXiv:1203.3551. Bibcode:2011ARNPS..61...47F. doi:10.1146/annurev-nucl-102010-130445. S2CID 119265528.
  59. Platts, E.; Weltman, A.; Walters, A.; Tendulkar, S.P.; Gordin, J.E.B.; Kandhai, S. (2019). "A living theory catalogue for fast radio bursts". Physics Reports. 821: 1–27. arXiv:1810.05836. Bibcode:2019PhR...821....1P. doi:10.1016/j.physrep.2019.06.003. S2CID 119091423.
  60. Schlein, Benjamin. "Graduate Seminar on Partial Differential Equations in the Sciences – Energy and Dynamics of Boson Systems". Hausdorff Center for Mathematics. Retrieved 23 April 2012.
  61. Barton, G.; Scharnhorst, K. (1993). "QED between parallel mirrors: light signals faster than c, or amplified by the vacuum". Journal of Physics A. 26 (8): 2037. Bibcode:1993JPhA...26.2037B. doi:10.1088/0305-4470/26/8/024. A more recent follow-up paper is Scharnhorst, K. (1998). "The velocities of light in modified QED vacua". Annalen der Physik. 7 (7–8): 700–709. arXiv:hep-th/9810221. Bibcode:1998AnP...510..700S. doi:10.1002/(SICI)1521-3889(199812)7:7/8<700::AID-ANDP700>3.0.CO;2-K.
  62. Kenneth Chang (29 July 2008). "The Nature of Glass Remains Anything but Clear". The New York Times.
  63. P.W. Anderson (1995). "Through the Glass Lightly". Science. 267 (5204): 1615–1616. doi:10.1126/science.267.5204.1615-e. PMID 17808155. S2CID 28052338. The deepest and most interesting unsolved problem in solid state theory is probably the theory of the nature of glass and the glass transition.
  64. Cryogenic electron emission phenomenon has no known physics explanation. Physorg.com. Retrieved on 20 October 2011.
  65. Meyer, H. O. (1 March 2010). "Spontaneous electron emission from a cold surface". Europhysics Letters. 89 (5): 58001. Bibcode:2010EL.....8958001M. doi:10.1209/0295-5075/89/58001.
  66. Storey, B. D.; Szeri, A. J. (8 July 2000). "Water vapour, sonoluminescence and sonochemistry". Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences. 456 (1999): 1685–1709. Bibcode:2000RSPSA.456.1685D. doi:10.1098/rspa.2000.0582. S2CID 55030028.
  67. Wu, C. C.; Roberts, P. H. (9 May 1994). "A Model of Sonoluminescence". Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences. 445 (1924): 323–349. Bibcode:1994RSPSA.445..323W. doi:10.1098/rspa.1994.0064. S2CID 122823755.
  68. Yoshida, Beni (1 October 2011). "Feasibility of self-correcting quantum memory and thermal stability of topological order". Annals of Physics. 326 (10): 2566–2633. arXiv:1103.1885. Bibcode:2011AnPhy.326.2566Y. doi:10.1016/j.aop.2011.06.001. ISSN 0003-4916. S2CID 119611494.
  69. Dean, Cory R. (2015). "Even denominators in odd places". Nature Physics. 11 (4): 298–299. Bibcode:2015NatPh..11..298D. doi:10.1038/nphys3298. ISSN 1745-2481.
  70. Mukherjee, Prabir K. (1998). "Landau Theory of Nematic-Smectic-A Transition in a Liquid Crystal Mixture". Molecular Crystals & Liquid Crystals. 312: 157–164. doi:10.1080/10587259808042438.
  71. A. Yethiraj, "Recent Experimental Developments at the Nematic to Smectic-A Liquid Crystal Phase Transition", Thermotropic Liquid Crystals: Recent Advances, ed. A. Ramamoorthy, Springer 2007, chapter 8.
  72. Norris, David J. (2003). "The Problem Swept Under the Rug". In Klimov, Victor (ed.). Electronic Structure in Semiconductors Nanocrystals: Optical Experiment (in Semiconductor and Metal Nanocrystals: Synthesis and Electronic and Optical Properties). CRC Press. p. 97. ISBN 978-0-203-91326-0.
  73. Lipa, J. A.; Nissen, J. A.; Stricker, D. A.; Swanson, D. R.; Chui, T. C. P. (14 November 2003). "Specific heat of liquid helium in zero gravity very near the lambda point". Physical Review B. 68 (17): 174518. arXiv:cond-mat/0310163. Bibcode:2003PhRvB..68q4518L. doi:10.1103/PhysRevB.68.174518. S2CID 55646571.
  74. Campostrini, Massimo; Hasenbusch, Martin; Pelissetto, Andrea; Vicari, Ettore (6 October 2006). "Theoretical estimates of the critical exponents of the superfluid transition in $^{4}\mathrm{He}$ by lattice methods". Physical Review B. 74 (14): 144506. arXiv:cond-mat/0605083. doi:10.1103/PhysRevB.74.144506. S2CID 118924734.
  75. Hasenbusch, Martin (26 December 2019). "Monte Carlo study of an improved clock model in three dimensions". Physical Review B. 100 (22): 224517. arXiv:1910.05916. Bibcode:2019PhRvB.100v4517H. doi:10.1103/PhysRevB.100.224517. ISSN 2469-9950. S2CID 204509042.
  76. Chester, Shai M.; Landry, Walter; Liu, Junyu; Poland, David; Simmons-Duffin, David; Su, Ning; Vichi, Alessandro (2020). "Carving out OPE space and precise $O(2)$ model critical exponents". Journal of High Energy Physics. 2020 (6): 142. arXiv:1912.03324. Bibcode:2020JHEP...06..142C. doi:10.1007/JHEP06(2020)142. S2CID 208910721.
  77. Rychkov, Slava (31 January 2020). "Conformal bootstrap and the λ-point specific heat experimental anomaly". Journal Club for Condensed Matter Physics. doi:10.36471/JCCM_January_2020_02.
  78. F. Wagner (2007). "A quarter-century of H-mode studies" (PDF). Plasma Physics and Controlled Fusion. 49 (12B): B1. Bibcode:2007PPCF...49....1W. doi:10.1088/0741-3335/49/12B/S01. S2CID 498401..
  79. André Balogh; Rudolf A. Treumann (2013). "Section 7.4 The Injection Problem". Physics of Collisionless Shocks: Space Plasma Shock Waves. p. 362. ISBN 978-1-4614-6099-2.
  80. Goldstein, Melvyn L. (2001). "Major Unsolved Problems in Space Plasma Physics". Astrophysics and Space Science. 277 (1/2): 349–369. Bibcode:2001Ap&SS.277..349G. doi:10.1023/A:1012264131485. S2CID 189821322.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.