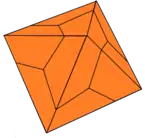
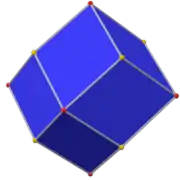
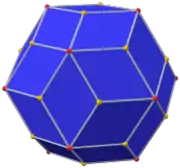
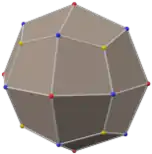
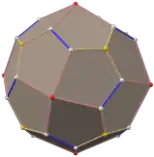
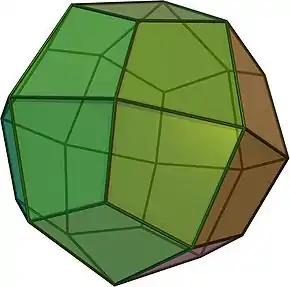
Deltoidal icositetrahedron
In geometry, a deltoidal icositetrahedron (also a trapezoidal icositetrahedron, tetragonal icosikaitetrahedron,[1] tetragonal trisoctahedron[2] and strombic icositetrahedron) is a Catalan solid. Its dual polyhedron is the rhombicuboctahedron.
| Deltoidal icositetrahedron | |
|---|---|
 (rotating and 3D model) | |
| Type | Catalan |
| Conway notation | oC or deC |
| Coxeter diagram | |
| Face polygon |  kite |
| Faces | 24 |
| Edges | 48 |
| Vertices | 26 = 6 + 8 + 12 |
| Face configuration | V3.4.4.4 |
| Symmetry group | Oh, BC3, [4,3], *432 |
| Rotation group | O, [4,3]+, (432) |
| Dihedral angle | 138°07′05″ arccos(−7 + 4√2/17) |
| Dual polyhedron | rhombicuboctahedron |
| Properties | convex, face-transitive |
 Net | |
Cartesian coordinates
Cartesian coordinates for a suitably sized deltoidal icositetrahedron centered at the origin are:
- (±1, 0, 0), (0, ±1, 0), (0, 0, ±1)
- (0, ±1/2√2, ±1/2√2), (±1/2√2, 0, ±1/2√2), (±1/2√2, ±1/2√2, 0)
- (±(2√2+1)/7, ±(2√2+1)/7, ±(2√2+1)/7)
The long edges of this deltoidal icosahedron have length √(2-√2) ≈ 0.765367.
Dimensions
The 24 faces are kites.[3] The short and long edges of each kite are in the ratio 1:(2 − 1/√2) ≈ 1:1.292893... If its smallest edges have length a, its surface area and volume are
The kites have three equal acute angles with value and one obtuse angle (between the short edges) with value .
Occurrences in nature and culture
The deltoidal icositetrahedron is a crystal habit often formed by the mineral analcime and occasionally garnet. The shape is often called a trapezohedron in mineral contexts, although in solid geometry that name has another meaning.
Orthogonal projections
The deltoidal icositetrahedron has three symmetry positions, all centered on vertices:
| Projective symmetry |
[2] | [4] | [6] |
|---|---|---|---|
| Image |  |
 |
 |
| Dual image |
 |
 |
 |
Related polyhedra
The solid's projection onto a cube divides its squares into quadrants. The projection onto an octahedron divides its triangles into kite faces. In Conway polyhedron notation this represents an ortho operation to a cube or octahedron.
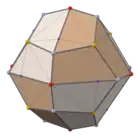
The solid (dual of the small rhombicuboctahedron) is similar to the disdyakis dodecahedron (dual of the great rhombicuboctahedron).
The main difference is, that the latter also has edges between the vertices on 3- and 4-fold symmetry axes (between yellow and red vertices in the images below).
 |
 |
 |
.png.webp) |
| Deltoidal icositetrahedron |
Disdyakis dodecahedron |
Dyakis dodecahedron |
Tetartoid |
Dyakis dodecahedron
A variant with pyritohedral symmetry is called a dyakis dodecahedron[4][5] or diploid.[6] It is common in crystallography.
It can be created by enlarging 24 of the 48 faces of the disdyakis dodecahedron. The tetartoid can be created by enlarging 12 of its 24 faces. [7]
Stellation
The great triakis octahedron is a stellation of the deltoidal icositetrahedron.
Related polyhedra and tilings
The deltoidal icositetrahedron is one of a family of duals to the uniform polyhedra related to the cube and regular octahedron.
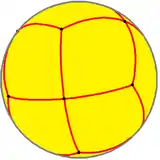
When projected onto a sphere (see right), it can be seen that the edges make up the edges of an octahedron and cube arranged in their dual positions. It can also be seen that the threefold corners and the fourfold corners can be made to have the same distance to the center. In that case the resulting icositetrahedron will no longer have a rhombicuboctahedron for a dual, since for the rhombicuboctahedron the centers of its squares and its triangles are at different distances from the center.
| Uniform octahedral polyhedra | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [4,3], (*432) | [4,3]+ (432) |
[1+,4,3] = [3,3] (*332) |
[3+,4] (3*2) | |||||||
| {4,3} | t{4,3} | r{4,3} r{31,1} |
t{3,4} t{31,1} |
{3,4} {31,1} |
rr{4,3} s2{3,4} |
tr{4,3} | sr{4,3} | h{4,3} {3,3} |
h2{4,3} t{3,3} |
s{3,4} s{31,1} |
= |
= |
= |
||||||||
| Duals to uniform polyhedra | ||||||||||
| V43 | V3.82 | V(3.4)2 | V4.62 | V34 | V3.43 | V4.6.8 | V34.4 | V33 | V3.62 | V35 |
This polyhedron is topologically related as a part of sequence of deltoidal polyhedra with face figure (V3.4.n.4), and continues as tilings of the hyperbolic plane. These face-transitive figures have (*n32) reflectional symmetry.
| Symmetry *n32 [n,3] |
Spherical | Euclid. | Compact hyperb. | Paraco. | ||||
|---|---|---|---|---|---|---|---|---|
| *232 [2,3] |
*332 [3,3] |
*432 [4,3] |
*532 [5,3] |
*632 [6,3] |
*732 [7,3] |
*832 [8,3]... |
*∞32 [∞,3] | |
| Figure Config. |
 V3.4.2.4 |
 V3.4.3.4 |
 V3.4.4.4 |
 V3.4.5.4 |
 V3.4.6.4 |
 V3.4.7.4 |
 V3.4.8.4 |
 V3.4.∞.4 |
See also
- Deltoidal hexecontahedron
- Tetrakis hexahedron, another 24-face Catalan solid which looks a bit like an overinflated cube.
- "The Haunter of the Dark", a story by H.P. Lovecraft, whose plot involves this figure
- Pseudo-deltoidal icositetrahedron
References
- Conway, Symmetries of Things, p.284–286
- https://etc.usf.edu/clipart/keyword/forms
- "Kite". Retrieved 6 October 2019.
- Isohedron 24k
- The Isometric Crystal System
- The 48 Special Crystal Forms
- Both is indicated in the two crystal models in the top right corner of this photo. A visual demonstration can be seen here and here.
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-9)
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, doi:10.1017/CBO9780511569371, ISBN 978-0-521-54325-5, MR 0730208 (The thirteen semiregular convex polyhedra and their duals, Page 23, Deltoidal icositetrahedron)
- The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 286, tetragonal icosikaitetrahedron)
External links
- Eric W. Weisstein, Deltoidal icositetrahedron (Catalan solid) at MathWorld.
- Deltoidal (Trapezoidal) Icositetrahedron – Interactive Polyhedron model




_-Krantz_374-.jpg.webp)