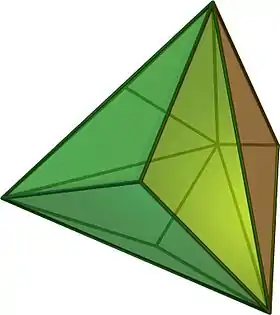
Triakis tetrahedron
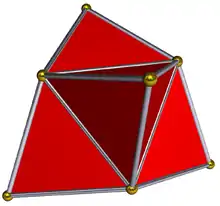
In geometry, a triakis tetrahedron (or kistetrahedron[1]) is a Catalan solid with 12 faces. Each Catalan solid is the dual of an Archimedean solid. The dual of the triakis tetrahedron is the truncated tetrahedron.
| Triakis tetrahedron | |
|---|---|
 (Click here for rotating model) | |
| Type | Catalan solid |
| Coxeter diagram | |
| Conway notation | kT |
| Face type | V3.6.6 isosceles triangle |
| Faces | 12 |
| Edges | 18 |
| Vertices | 8 |
| Vertices by type | 4{3}+4{6} |
| Symmetry group | Td, A3, [3,3], (*332) |
| Rotation group | T, [3,3]+, (332) |
| Dihedral angle | 129°31′16″ arccos(−7/11) |
| Properties | convex, face-transitive |
 Truncated tetrahedron (dual polyhedron) |
 Net |

The triakis tetrahedron can be seen as a tetrahedron with a triangular pyramid added to each face; that is, it is the Kleetope of the tetrahedron. It is very similar to the net for the 5-cell, as the net for a tetrahedron is a triangle with other triangles added to each edge, the net for the 5-cell a tetrahedron with pyramids attached to each face. This interpretation is expressed in the name.
The length of the shorter edges is 3/5 that of the longer edges.[2] If the triakis tetrahedron has shorter edge length 1, it has area 5/3√11 and volume 25/36√2.
Cartesian coordinates
Cartesian coordinates for the 8 vertices of a triakis tetrahedron centered at the origin, are the points (±5/3, ±5/3, ±5/3) with an even number of minus signs, along with the points (±1, ±1, ±1) with an odd number of minus signs:
- (5/3, 5/3, 5/3), (5/3, -5/3, -5/3), (-5/3, 5/3, -5/3), (-5/3, -5/3, 5/3)
- (-1, 1, 1), (1, -1, 1), (1, 1, -1), (-1, -1, -1)
The length of the shorter edges of this triakis tetrahedron equals . The faces are isosceles triangles with one obtuse and two acute angles. The obtuse angle equals and the acute ones equal .
Tetartoid symmetry
The triakis tetrahedron can be made as a degenerate limit of a tetartoid:
 |
 |
 |
 |
 |
 |
 |
 |
Orthogonal projections
| Centered by | Edge normal | Face normal | Face/vertex | Edge |
|---|---|---|---|---|
| Triakis tetrahedron |
 |
 |
 |
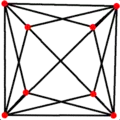 |
| (Dual) Truncated tetrahedron |
 |
 |
 |
 |
| Projective symmetry |
[1] | [1] | [3] | [4] |
Variations
A triakis tetrahedron with equilateral triangle faces represents a net of the four-dimensional regular polytope known as the 5-cell.
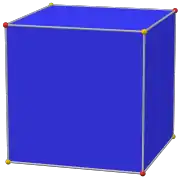
If the triangles are right-angled isosceles, the faces will be coplanar and form a cubic volume. This can be seen by adding the 6 edges of tetrahedron inside of a cube.
Related polyhedra
The triakis tetrahedron is a part of a sequence of polyhedra and tilings, extending into the hyperbolic plane. These face-transitive figures have (*n32) reflectional symmetry.
| *n32 symmetry mutation of truncated tilings: t{n,3} | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *n32 [n,3] |
Spherical | Euclid. | Compact hyperb. | Paraco. | Noncompact hyperbolic | ||||||
| *232 [2,3] |
*332 [3,3] |
*432 [4,3] |
*532 [5,3] |
*632 [6,3] |
*732 [7,3] |
*832 [8,3]... |
*∞32 [∞,3] |
[12i,3] | [9i,3] | [6i,3] | |
| Truncated figures |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 | |
| Symbol | t{2,3} | t{3,3} | t{4,3} | t{5,3} | t{6,3} | t{7,3} | t{8,3} | t{∞,3} | t{12i,3} | t{9i,3} | t{6i,3} |
| Triakis figures |
 |
 |
 |
 |
 |
 |
 |
||||
| Config. | V3.4.4 | V3.6.6 | V3.8.8 | V3.10.10 | V3.12.12 | V3.14.14 | V3.16.16 | V3.∞.∞ | |||
| Family of uniform tetrahedral polyhedra | |||||||
|---|---|---|---|---|---|---|---|
| Symmetry: [3,3], (*332) | [3,3]+, (332) | ||||||
 |
 |
 |
 |
||||
| {3,3} | t{3,3} | r{3,3} | t{3,3} | {3,3} | rr{3,3} | tr{3,3} | sr{3,3} |
| Duals to uniform polyhedra | |||||||
 |
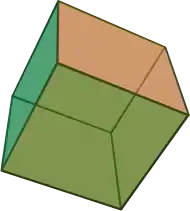 |
 |
 | ||||
| V3.3.3 | V3.6.6 | V3.3.3.3 | V3.6.6 | V3.3.3 | V3.4.3.4 | V4.6.6 | V3.3.3.3.3 |
See also
References
- Conway, Symmetries of things, p.284
- https://rechneronline.de/pi/triakis-tetrahedron.php
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-9)
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, doi:10.1017/CBO9780511569371, ISBN 978-0-521-54325-5, MR 0730208 (The thirteen semiregular convex polyhedra and their duals, Page 14, Triakistetrahedron)
- The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 284, Triakis tetrahedron )