Ionization energy

In physics and chemistry, ionization energy (American English spelling) or ionisation energy (British English spelling) is the minimum amount of energy required to remove the most loosely bound electron of an isolated neutral gaseous atom or molecule.[1] It is quantitatively expressed as

X(g) + energy ⟶ X+(g) + e−
For the values of the ionization energies of the elements, see Molar ionization energies of the elements and Ionization energies of the elements (data page)
Ionization energy trends plotted against the atomic number. The ionization energy gradually increases from the alkali metals to the noble gases. The maximum ionization energy also decreases from the first to the last row in a given column, due to the increasing distance of the valence electron shell from the nucleus.

where X is any atom or molecule, X+ is the ion with one electron removed, and e− is the removed electron.[2] This is generally an endothermic process. As a rule, the closer the outermost electrons to the nucleus of the atom, the higher the atom's ionization energy.

The sciences of physics and chemistry use different units for ionization energy.[3] In physics, the unit is the amount of energy required to remove a single electron from a single atom or molecule, expressed as electronvolts. In chemistry, the unit is the amount of energy required for all of the atoms in a mole of substance to lose one electron each: molar ionization energy or approximately enthalpy, expressed as kilojoules per mole (kJ/mol) or kilocalories per mole (kcal/mol).[4]

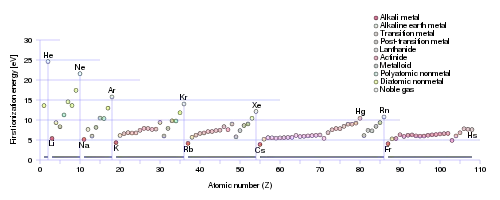
Comparison of ionization energies of atoms in the periodic table reveals two periodic trends which follow the rules of Coulombic attraction:[5]

  1. Ionization energy generally increases from left to right within a given period (that is, row).
  2. Ionization energy generally decreases from top to bottom in a given group (that is, column).

The latter trend results from the outer electron shell being progressively farther from the nucleus, with the addition of one inner shell per row as one moves down the column.

The nth ionization energy refers to the amount of energy required to remove an electron from the species having a charge of (n-1). For example, the first three ionization energies are defined as follows:

1st ionization energy is the energy that enables the reaction X ⟶ X+ + e−
2nd ionization energy is the energy that enables the reaction X+ ⟶ X2+ + e−
3rd ionization energy is the energy that enables the reaction X2+ ⟶ X3+ + e−

The term ionization potential is an older and obsolete term[6] for ionization energy,[7] because the oldest method of measuring ionization energy was based on ionizing a sample and accelerating the electron removed using an electrostatic potential.

The most notable factors affecting the ionization energy include:

  • Electron configuration: this accounts for most element's IE, as all of their chemical and physical characteristics can be ascertained just by determining their respective electron configuration.
  • Nuclear charge: if the nuclear charge (atomic number) is greater, the electrons are held more tightly by the nucleus and hence the ionization energy will be greater.
  • Number of electron shells: if the size of the atom is greater due to the presence of more shells, the electrons are held less tightly by the nucleus and the ionization energy will be lesser.
  • Effective nuclear charge (Zeff): if the magnitude of electron shielding and penetration are greater, the electrons are held less tightly by the nucleus, the Zeff of the electron and the ionization energy is lesser.[8]
  • Type of orbital ionized: an atom having a more stable electronic configuration has less tendency to lose electrons and consequently has higher ionization energy.
  • Electron occupancy: if the highest occupied orbital is doubly occupied, then it is easier to remove an electron.

Other minor factors include:

  • Relativistic effects: heavier elements (especially those whose atomic number is greater than 70) are affected by these as their electrons are approaching the speed of light, and hence have a smaller atomic radius/higher IE.
  • Lanthanide and actinide contraction (and scandide contraction): the unprecedented shrinking of the elements affect the ionization energy, as the net charge of the nucleus is more strongly felt.
  • Electron pair energies and exchange energy: these would only account for fully filled and half-filled orbitals. A common misconception is that "symmetry" plays a part; albeit, none so far has concluded its evidence.

Determination of ionization energies
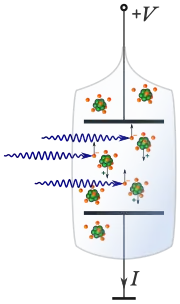
Ionization energy measurement apparatus.

Ionization energy of atoms, denoted Ei, is measured[9] by finding the minimal energy of light quanta (photons) or electrons accelerated to a known energy that will kick out the least bound atomic electrons. The measurement is performed in the gas phase on single atoms. While only noble gases occur as monoatomic gases, other gases can be split into single atoms. Also, many solid elements can be heated and vaporized into single atoms. Monoatomic vapor is contained in a previously evacuated tube that has two parallel electrodes connected to a voltage source. The ionizing excitation is introduced through the walls of the tube or produced within.

When ultraviolet light is used, the wavelength is swept down the ultraviolet range. At a certain wavelength (λ) and frequency of light (ν=c/λ, where c is the speed of light), the light quanta, whose energy is proportional to the frequency, will have energy high enough to dislodge the least bound electrons. These electrons will be attracted to the positive electrode, and the positive ions remaining after the photoionization will get attracted to the negatively charged electrode. These electrons and ions will establish a current through the tube. The ionization energy will be the energy of photons hνi (h is the Planck constant) that caused a steep rise in the current: Ei=hνi.

When high-velocity electrons are used to ionize the atoms, they are produced by an electron gun inside a similar evacuated tube. The energy of the electron beam can be controlled by the acceleration voltages. The energy of these electrons that gives rise to a sharp onset of the current of ions and freed electrons through the tube will match the ionization energy of the atoms.

Generally, the (n+1)th ionization energy of a particular element is larger than the nth ionization energy. When the next ionization energy involves removing an electron from the same electron shell, the increase in ionization energy is primarily due to the increased net charge of the ion from which the electron is being removed. Electrons removed from more highly charged ions experience greater forces of electrostatic attraction; thus, their removal requires more energy. In addition, when the next ionization energy involves removing an electron from a lower electron shell, the greatly decreased distance between the nucleus and the electron also increases both the electrostatic force and the distance over which that force must be overcome to remove the electron. Both of these factors further increase the ionization energy.

Some values for elements of the third period are given in the following table:

Successive ionization energy values / kJ mol−1
(96.485 kJ mol−1 ≡ 1 eV)
Element First Second Third Fourth Fifth Sixth Seventh
Na 496 4,560
Mg 738 1,450 7,730
Al 577 1,816 2,881 11,600
Si 786 1,577 3,228 4,354 16,100
P 1,060 1,890 2,905 4,950 6,270 21,200
S 1,000 2,295 3,375 4,565 6,950 8,490 27,107
Cl 1,256 2,260 3,850 5,160 6,560 9,360 11,000
Ar 1,520 2,665 3,945 5,770 7,230 8,780 12,000

Large jumps in the successive molar ionization energies occur when passing noble gas configurations. For example, as can be seen in the table above, the first two molar ionization energies of magnesium (stripping the two 3s electrons from a magnesium atom) are much smaller than the third, which requires stripping off a 2p electron from the neon configuration of Mg2+. That electron is much closer to the nucleus than the 3s electron removed previously.

Ionization energies peak in noble gases at the end of each period in the periodic table of elements and, as a rule, dip when a new orbital is starting to be filled.

Ionization energy is also a periodic trend within the periodic table. Moving left to right within a period, or upward within a group, the first ionization energy generally increases,[10] with exceptions such as aluminium and sulfur in the table above. As the nuclear charge of the nucleus increases across the period, the electron shielding remains constant, hence the atomic radius decreases, and the electron cloud becomes closer towards the nucleus[11] because the electrons, especially the outermost one, are held tighter by the higher effective nuclear charge. Similarly on moving upward within a given group, the electrons are held in lower-energy orbitals, closer to the nucleus and therefore are more tightly bound.[12]

Exceptions in ionization energies

There are exceptions to the general trend of rising ionization energies within a period. For example, the value decreases from beryllium ( 
4Be
: 9.3 eV) to boron ( 
5B
: 8.3 eV), and from nitrogen ( 
7N
: 14.5 eV) to oxygen ( 
8O
: 13.6 eV). These dips can be explained in terms of electron configurations.[13]

The added electron in boron occupies a p-orbital.

Boron has its last electron in a 2p orbital, which has its electron density further away from the nucleus on average than the 2s electrons in the same shell. The 2s electrons then shield the 2p electron from the nucleus to some extent, and it is easier to remove the 2p electron from boron than to remove a 2s electron from beryllium, resulting in lower ionization energy for B.[2]

These electron configurations do not show the full and half-filled orbitals.
Here the added electron has a spin opposed to the other 2p electrons. This decreases the ionization energy of oxygen

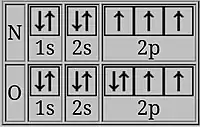
In oxygen, the last electron shares a doubly occupied p-orbital with an electron of opposing spin. The two electrons in the same orbital are closer together on average than two electrons in different orbitals, so that they shield each other more effectively and it is easier to remove one, resulting in lower ionization energy.[2][14]

Furthermore, after every noble gas element, the ionization energy drastically drops. This occurs because the outer electron in the alkali metals requires a much lower amount of energy to be removed from the atom than the inner shells. This also gives rise to low electronegativity values for the alkali metals.[15][16][17]

Because of a single p-orbital electron in gallium's configuration, makes the overall structure less stable, hence the dip in ionization energy values[18]
Actinium's electron configuration predetermines that it would require less energy in order to remove that single f-orbital electron, ergo even though it has a larger EC, radium still has the higher IE[19]

The trends and exceptions are summarized in the following subsections:

Ionization energy decreases when:
  • Transitioning to a new period: an alkali metal easily loses one electron to leave an octet or pseudo-noble gas configuration, so those elements have only small values for IE.
  • Moving from the s-block to the p-block: a p-orbital loses an electron more easily. An example is beryllium to boron, with electron configuration 1s2 2s2 2p1. The 2s electrons shield the higher-energy 2p electron from the nucleus, making it slightly easier to remove. This also happens in magnesium to aluminum.[20]
  • Occupying a p-subshell with its first electron with spin opposed to the other electrons: such as in nitrogen ( 
    7    N
    : 14.5 eV) to oxygen ( 
    8    O
    : 13.6 eV), as well as phosphorus ( 
    15    P
    : 10.48 eV) to sulfur ( 
    16    S
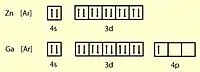
    : 10.36 eV). The reason for this is because oxygen, sulfur and selenium all have dipping ionization energies because of shielding effects.[21] However, this discontinues starting from tellurium where the shielding is too small to produce a dip.
  • Moving from the d-block to the p-block: as in the case of zinc ( 
    30    Zn
    : 9.4 eV) to gallium ( 
    31    Ga
    : 6.0 eV)
  • Special case: decrease from lead ( 
    82    Pb
    : 7.42 eV) to bismuth ( 
    83    Bi
    : 7.29 eV). This cannot be attributed to size (the difference is minimal: lead has a covalent radius of 146 pm whereas bismuth's is 148 pm[22]). Nor can it be attributed to relativistic stabilization of the 6s orbital, as this factor is very similar in the two adjacent elements. Other factors suggest contrary to fact that bismuth should have the higher IE, due to its half-filled orbital (adding stabilization), position in the periodic table (Bi is further right so it should be less metallic than Pb), and it has one more proton (contributes to the [effective] nuclear charge).[23]
  • Special case: decrease from radium ( 
    88    Ra
    : 5.27 eV) to actinium ( 
    89    Ac
    : 5.17 eV) which is a switch from a p to an f orbital. However the analogous switch from barium ( 
    56    Ba
    : 5.2 eV) to lanthanum ( 
    57    La
    : 5.6 eV) does not show a downward change.
  • Lutetium ( 
    71    Lu
    ) and lawrencium ( 
    103    Lr
    ) both have ionization energies lower than the previous elements. In both cases the last electron added starts a new subshell: 5d for Lu with electron configuration [Xe] 4f14 5d1 6s2, and 7p for Lr with configuration [Rn] 5f4 7s2 7p1. These dips in ionization energies have since been used as evidence in the ongoing debate over whether Lu and Lr should be placed in Group 3 of the periodic table instead of lanthanum (La) and actinium (Ac).[24][25] [26][27]

Ionization energy increases when:
  • Reaching Group 18 Noble gas elements: This is due to their complete electron subshells,[28] so that these elements require large amounts of energy to remove one electron.
  • Group 12: The elements here, zinc ( 
    30    Zn
    : 9.4 eV), cadmium ( 
    48    Cd
    : 9.0 eV) and mercury ( 
    80    Hg
    : 10.4 eV) all record sudden rising IE values in contrast to their preceding elements: copper ( 
    29    Cu
    : 7.7 eV), silver ( 
    47    Ag
    : 7.6 eV) and gold ( 
    79    Au
    : 9.2 eV) respectively. For mercury, it can be extrapolated that the relativistic stabilization of the 6s electrons increases the ionization energy, in addition to poor shielding by 4f electrons that increases the effective nuclear charge on the outer valence electrons. In addition, the closed-subshells electron configurations: [Ar] 3d10 4s2, [Kr] 4d105s2 and [Xe] 4f14 5d10 6s2 provide increased stability.
  • Special case: shift from rhodium ( 
    45    Rh
    : 7.5 eV) to palladium ( 
    46    Pd
    : 8.3 eV). Unlike other Group 10 elements, palladium has a higher ionization energy than the preceding atom, due to its electron configuration. In contrast to nickel's [Ar] 3d8 4s2, and platinum's [Xe] 4f14 5d9 6s1, palladium's electron configuration is [Kr] 4d10 5s0 (even though the Madelung rule predicts [Kr] 4d8 5s2). Finally, silver's lower IE ( 
    47    Ag
    : 7.6 eV) further accentuates the high value for palladium; the single added s electron is removed with a lower ionization energy than palladium,[29] which emphasizes palladium's high IE (as shown in the above linear table values for IE)
  • The IE of gadolinium ( 
    64    Gd
    : 6.15 eV) is somewhat higher than both the preceding ( 
    62    Sm
    : 5.64 eV), ( 
    63    Eu
    : 5.67 eV) and following elements ( 
    65    Tb
    : 5.86 eV), ( 
    66    Dy
    : 5.94 eV). This anomaly may be attributed to the half-filled 4f7 orbital.
  • Moving into d-block elements: The elements Sc with a 3d1 electronic configuration has a higher IP ( 
    21    Sc
    : 6.56 eV) than the preceding element ( 
    20    Ca
    : 6.11 eV), contrary to the decreases on moving into s-block and p-block elements. The 4s and 3d electrons have similar shielding ability: the 3d orbital forms part of the n=3 shell whose average position is closer to the nucleus than the 4s orbital and the n=4 shell, but electrons in s orbitals experience greater penetration into the nucleus than electrons in d orbitals. So the mutual shielding of 3d and 4s electrons is weak, and the effective nuclear charge acting on the ionized electron is relatively large. Yttrium ( 
    39    Y
    ) similarly has a higher IP (6.22 eV) than  
    38    Sr
    : 5.69 eV. The last two d1 elements ( 
    57    La
    : 5.18 eV) and ( 
    89    Ac
    : 5.17 eV) have only very slightly lower IP's than their preceding elements ( 
    56    Ba
    : 5.21 eV) and ( 
    88    Ra
    : 5.18 eV).
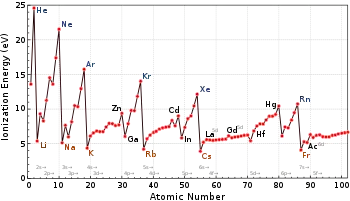
  • Moving into f-block elements; As can be seen in the above graph for ionization energies, the sharp rise in IE values from ( 
    55    Cs
    ) to ( 
    57    La
    ) is followed by a small almost linear increase as f electrons are added. This is due to the lanthanide contraction (for lanthanides).[30][31][32] This decrease in ionic radius is associated with an increase in ionization energy in turn increases, since the two properties correlate to each other.[10] As for d-block elements, the electrons are added in an inner shell, so that no new shells are formed. The shape of the added orbitals prevents them from penetrating to the nucleus so that the electrons occupying them have less shielding capacity.

Ionization energy anomalies in groups

Ionization energy values tend to decrease on going to heavier elements within a group[13] as shielding is provided by more electrons and overall, the valence shells experience experience a weaker attraction from the nucleus.[12](attributed to the larger covalent radius which increase on going down a group[33]) Nonetheless, this isn't always the case. As one exception, in Group 10 palladium ( 
46Pd
: 8.34 eV) has a higher ionization energy than nickel ( 
28Ni
: 7.64 eV), contrary to the general decrease for the elements from technetium  
43Tc
 to xenon  
54Xe
. Such anomalies are summarized below:

  • Group 1:
    • Hydrogen's ionization energy is very high (at 13.59844 eV), compared to the alkali metals. This is due to its single electron (and hence, very small electron cloud), which is close to the nucleus. Likewise, since there aren't any other electrons that may cause shielding, that single electron experiences the full net positive charge of the nucleus.[34]
    • Francium's ionization energy is higher than the precedent alkali metal, cesium. This is due to its (and radium's) small ionic radii owing to relativistic effects. Because of their large mass and size, this means that its electrons are traveling at extremely high speeds which results in the electrons become closer to the nucleus than expected, and they are consequently harder to remove (higher IE).[35]
  • Group 2: Radium's ionization energy which is higher than its antecedent alkaline earth metal barium, like francium, is also due to relativistic effects. The electrons, especially the 1s electrons, experience very high effective nuclear charges. To avoid falling into the nucleus, the 1s electrons must orbit at very high speeds, which causes the special relativistic corrections to be substantially higher than the approximate classical momenta. By the uncertainty principle, this causes a relativistic contraction of the 1s orbital (and other orbitals with electron density close to the nucleus, especially ns and np orbitals). Hence this causes a cascade of electron changes which finally results in the outermost electron shells contracting and getting closer to the nucleus.
  • Group 14:Lead's ( 
    82    Pb
    : 7.4 eV) unusually high ionization energy.This is because of the inclusion not only of the 5d electrons but also the 4f electrons (the lanthanides). The 4f electrons screen the nucleus rather inefficiently from 6p electrons causing the effective nuclear charge to be quite high, to the extent that the ionization energy for lead is actually a little higher than that of tin.[36]
  • Group 4:
    • Hafnium's near similarity in IE than zirconium. The effects of the lanthanide contraction can still be felt after the lanthanides.[31] It can be seen through the former's lesser atomic radii (which contradicts the observed periodic trend) at 159 pm[37] (empirical value) which differs from the latter's 155 pm.[38][39] This in turn makes its ionization energies increase by 18±kJ/mol−1.
      • Titanium's IE, which is lesser than both hafnium and zirconium. Hafnium's ionization energy is similar to zirconium due to lanthanide contraction. However, why zirconium's ionization energy is higher than the element preceding it remains shrouded; we cannot rule atomic radii as in fact it is higher for zirconium and hafnium by 15 pm.[40] We also cannot rule the condensed ionization energy, as they're more or less the same ([Ar] 3d² 4s² for titanium, whereas [Kr] 4d² 5s² for zirconium). Additionally, there are no half-filled nor fully filled orbitals we might compare. Hence, we can only rule out zirconium's full electron configuration, which is 1s²2s²2p⁶3s²3p⁶3d¹⁰4s²4p⁶4d²5s².[41] The presence of a full 3d-block sublevel is tantamount to a higher shielding efficiency compared to the 4d-block elements (which are only two electrons).[lower-alpha 1]
  • Group 5: akin to Group 4, niobium and tantalum are analogous to each other, due to their electron configuration and to the lanthanide contraction affecting the latter element.[42] Ipso facto, their significant rise in IE compared to the foremost element in the group, vanadium, can be attributed due to their full d-block electrons, in addition to their electron configuration. Another intriguing notion is niobium's half-filled 5s orbital; due to repulsion and exchange energy (in other words the "costs" for putting an electron in a low-energy sublevel to completely fill it instead of putting the electron in a high-energy one) overcoming the energy gap between s- and d-(or f) block electrons, the EC does not follow the Madelung rule.
  • Group 6: like its forerunners groups 4 and 5, group 6 also record high values when moving downward. Tungsten is once again similar to molybdenum due to their electron configurations.[43] Likewise, it is also attributed to the full 3d-orbital in its electron configuration. Another reason is molybdenum's half filled 4d orbital due to electron pair energies violating the aufbau principle.
  • Groups 7-12 6th period elements (rhenium, osmium, iridium, platinum, gold and mercury): All of these elements have extremely high ionization energies than the element preceding them in their respective groups. The essence of this is due to the lanthanide contraction's influence to post lanthanides, in addition to the relativistic stabilization of the 6s orbital.
  • Group 13:
    • Gallium's IE which is higher than aluminum. This is once again due to d-orbitals, in addition to scandide contraction, providing weak shielding, and hence the effective nuclear charges are augmented.
    • Thallium's IE, due to poor shielding of 4f electrons[44] in addition to lanthanide contraction, causes it's IE to be heightened in contrast to its precursor indium.
  • Group 14: Lead having a higher IE compared to tin. This is attributed to, akin to Group IIIA's thallium, poor shielding by f orbital and lanthanide contraction.[44]

Electrostatic explanation

Atomic ionization energy can be predicted by an analysis using electrostatic potential and the Bohr model of the atom, as follows (note that the derivation uses Gaussian units).

Consider an electron of charge -e and an atomic nucleus with charge +Ze, where Z is the number of protons in the nucleus. According to the Bohr model, if the electron were to approach and bond with the atom, it would come to rest at a certain radius a. The electrostatic potential V at distance a from the ionic nucleus, referenced to a point infinitely far away, is:

Since the electron is negatively charged, it is drawn inwards by this positive electrostatic potential. The energy required for the electron to "climb out" and leave the atom is:

This analysis is incomplete, as it leaves the distance a as an unknown variable. It can be made more rigorous by assigning to each electron of every chemical element a characteristic distance, chosen so that this relation agrees with experimental data.

It is possible to expand this model considerably by taking a semi-classical approach, in which momentum is quantized. This approach works very well for the hydrogen atom, which only has one electron. The magnitude of the angular momentum for a circular orbit is:

The total energy of the atom is the sum of the kinetic and potential energies, that is:

Velocity can be eliminated from the kinetic energy term by setting the Coulomb attraction equal to the centripetal force, giving:

Solving the angular momentum for v and substituting this into the expression for kinetic energy, we have:

This establishes the dependence of the radius on n. That is:

Now the energy can be found in terms of Z, e, and r. Using the new value for the kinetic energy in the total energy equation above, it is found that:

At its smallest value, n is equal to 1 and r is the Bohr radius a0 which equals . Now, the equation for the energy can be established in terms of the Bohr radius. Doing so gives the result:

Quantum-mechanical explanation

According to the more complete theory of quantum mechanics, the location of an electron is best described as a probability distribution within an electron cloud, i.e. atomic orbital.[45][46]The energy can be calculated by integrating over this cloud. The cloud's underlying mathematical representation is the wavefunction which is built from Slater determinants consisting of molecular spin orbitals. These are related by Pauli's exclusion principle to the antisymmetrized products of the atomic or molecular orbitals.

There are two main ways ionization energy is calculated. In general, the computation for the nth ionization energy requires calculating the energies of and electron systems. Calculating these energies exactly is not possible except for the simplest systems (i.e. hydrogen and hydrogen-like elements), primarily because of difficulties in integrating the electron correlation terms. Therefore, approximation methods are routinely employed, with different methods varying in complexity (computational time) and accuracy compared to empirical data. This has become a well-studied problem and is routinely done in computational chemistry. The second way of calculating ionization energies is mainly used at the lowest level of approximation, where the ionization energy is provided by Koopmans' theorem, which involves the highest occupied molecular orbital or "HOMO" and the lowest unoccupied molecular orbital or "LUMO", which states that the ionization energy of an atom or molecule is equal to the energy of the orbital from which the electron is ejected. This means that the ionization energy is equal to the HOMO energy, whose formal equation is equal to: .[47]

Vertical and adiabatic ionization energy in molecules
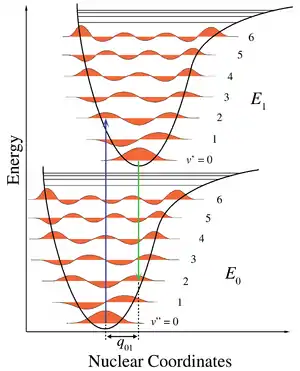
Figure 1. Franck–Condon principle energy diagram. For ionization of a diatomic molecule, the only nuclear coordinate is the bond length. The lower curve is the potential energy curve of the neutral molecule, and the upper curve is for the positive ion with a longer bond length. The blue arrow is vertical ionization, here from the ground state of the molecule to the v=2 level of the ion.

Ionization of molecules often leads to changes in molecular geometry, and two types of (first) ionization energy are defined – adiabatic and vertical.[48]

Adiabatic ionization energy

The adiabatic ionization energy of a molecule is the minimum amount of energy required to remove an electron from a neutral molecule, i.e. the difference between the energy of the vibrational ground state of the neutral species (v" = 0 level) and that of the positive ion (v' = 0). The specific equilibrium geometry of each species does not affect this value.

Vertical ionization energy

Due to the possible changes in molecular geometry that may result from ionization, additional transitions may exist between the vibrational ground state of the neutral species and vibrational excited states of the positive ion. In other words, ionization is accompanied by vibrational excitation. The intensity of such transitions is explained by the Franck–Condon principle, which predicts that the most probable and intense transition corresponds to the vibrationally excited state of the positive ion that has the same geometry as the neutral molecule. This transition is referred to as the "vertical" ionization energy since it is represented by a completely vertical line on a potential energy diagram (see Figure).

For a diatomic molecule, the geometry is defined by the length of a single bond. The removal of an electron from a bonding molecular orbital weakens the bond and increases the bond length. In Figure 1, the lower potential energy curve is for the neutral molecule and the upper surface is for the positive ion. Both curves plot the potential energy as a function of bond length. The horizontal lines correspond to vibrational levels with their associated vibrational wave functions. Since the ion has a weaker bond, it will have a longer bond length. This effect is represented by shifting the minimum of the potential energy curve to the right of the neutral species. The adiabatic ionization is the diagonal transition to the vibrational ground state of the ion. Vertical ionization may involve vibrational excitation of the ionic state and therefore requires greater energy.

In many circumstances, the adiabatic ionization energy is often a more interesting physical quantity since it describes the difference in energy between the two potential energy surfaces. However, due to experimental limitations, the adiabatic ionization energy is often difficult to determine, whereas the vertical detachment energy is easily identifiable and measurable.

Analogs of ionization energy to other systems

While the term ionization energy is largely used only for gas-phase atomic or molecular species, there are a number of analogous quantities that consider the amount of energy required to remove an electron from other physical systems.

Electron binding energy
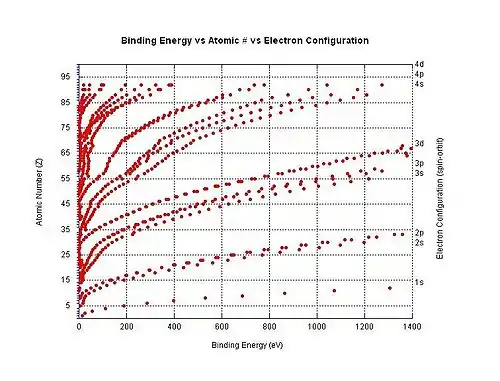
Binding energies of specific atomic orbitals as a function of the atomic number. Because of the increasing number of protons, electrons occupying the same orbital are more tightly bound in heavier elements.

Electron binding energy is a generic term for the minimum energy needed to remove an electron from a particular electron shell for an atom or ion, due to these negatively charged electrons being held in place by the electrostatic pull of the positively charged nucleus.[49] For example, the electron binding energy for removing a 3p3/2 electron from the chloride ion is the minimum amount of energy required to remove an electron from the chlorine atom when it has a charge of -1. In this particular example, the electron binding energy has the same magnitude as the electron affinity for the neutral chlorine atom. In another example, the electron binding energy refers to the minimum amount of energy required to remove an electron from the dicarboxylate dianion −O2C(CH2)8CO−
2.

The graph to the right shows the binding energy for electrons in different shells in neutral atoms. The ionization energy is the lowest binding energy for a particular atom (although these are not all shown in the graph).

Work function

Work function is the minimum amount of energy required to remove an electron from a solid surface, where the work function W for a given surface is defined by the difference[50]

where −e is the charge of an electron, ϕ is the electrostatic potential in the vacuum nearby the surface, and EF is the Fermi level (electrochemical potential of electrons) inside the material.

Note
  1. Nonetheless, further research is still needed to corroborate this mere inference.

See also
  • Rydberg equation - a calculation that could determine the ionization energies of hydrogen and hydrogen-like elements. This is further elaborated through this site.
  • Electron affinity – a closely related concept describing the energy released by adding an electron to a neutral atom or molecule.
  • Lattice energy — a measure of the energy released when ions are combined to make a compound.
  • Electronegativity is a number that shares some similarities with ionization energy.
  • Koopmans' theorem, regarding the predicted ionization energies in Hartree–Fock theory.
  • Di-tungsten tetra(hpp) has the lowest recorded ionization energy for a stable chemical compound.
  • Bond-dissociation energy- measure of the strength of a chemical bond calculated through cleaving by homolysis giving two radical fragments A and B and subsequent evaluation of the enthalpy change
  • Bond energy- the average measure of a chemical bond’s strength, calculated through the amount of heat needed to break all of the chemical bonds into individual atoms.

References
  1. "Periodic Trends". Chemistry LibreTexts. 2013-10-02. Retrieved 2020-09-13.
  2. Miessler, Gary L.; Tarr, Donald A. (1999). Inorganic Chemistry (2nd ed.). Prentice Hall. p. 41. ISBN 0-13-841891-8.
  3. The Editors, of Encyclopædia Britannica (May 29, 2020). "Ionization energy". britannica.com. Encyclopædia Britannica. Retrieved November 3, 2020.
  4. "Ionization Energy". ChemWiki. University of California, Davis. 2013-10-02.
  5. "Chapter 9: Quantum Mechanics". faculty.chem.queesu.ca. January 15, 2018. Retrieved October 31, 2020.
  6. "IUPAC - ionization potential (I03208)". goldbook.iupac.org. Retrieved 2020-09-13.
  7. Cotton, F. Albert; Wilkinson, Geoffrey (1988). Advanced Inorganic Chemistry (5th ed.). John Wiley. p. 1381. ISBN 0-471-84997-9.
  8. Lang, Peter F.; Smith, Barry C. (2003). "Ionization Energies of Atoms and Atomic Ions". Journal of Chemical Education. 80 (8): 938. Bibcode:2003JChEd..80..938L. doi:10.1021/ed080p938.
  9. Mahan, Bruce H. (1962). "Ionization Energy". College of Chemistry, University of California Berkeley. Retrieved 2020-09-13.
  10. Stone, E.G. (December 19, 2020v). "Atomic Structure : Periodic Trends". Department of Chemistry. chem.tamu.edu. 400 Bizzell St, College Station, TX 77843, Texas, United States: Texas A&M University. Retrieved December 19, 2020.CS1 maint: location (link)
  11. "Anomalous trends in ionization energy". Chemistry Stack Exchange. Retrieved 2020-09-20.
  12. "Ionization Energy | Introduction to Chemistry". courses.lumenlearning.com. Retrieved 2020-09-13.
  13. Grandinetti, Philip J. (September 8, 2019). "Ionization Energy Trends | Grandinetti Group". www.grandinetti.org. Retrieved 2020-09-13.
  14. Kent, Mr. "First Ionization Energy". kentchemistry.com. KentChemistry. Retrieved December 6, 2020. ...The addition of the second electron into an already occupied orbital introduces repulsion between the electrons, thus it is easier to remove. that is why there is a dip in the ionization energy.
  15. "Group IA". chemed.chem.purdue.edu. Retrieved 2020-09-20.
  16. "Alkali Metals". hyperphysics.phy-astr.gsu.edu. Retrieved 2020-09-13.
  17. "The Alkali Metals | Introduction to Chemistry". courses.lumenlearning.com. Retrieved 2020-09-13.
  18. Lang, P.F.; Smith, B.C. (August 1, 2003). Written at Birkbeck College (University of London), Malet Street, London WC1E 7HX, England, United Kingdom. "Ionization Energies of Atoms and Atomic Ions" (PDF). School of Biological and Chemical Sciences. Journal of Chemical Education. Washington, D.C., USA: ACS Publications. 80 (8): 938-946. doi:10.1021/ed080p938. ISBN 0-471-84997-9. ISSN 0021-9584. OCLC 223588922. S2CID 95492184. Retrieved December 19, 2020 – via Universidad de Valencia.CS1 maint: location (link) J. Chem. Educ. 2003, 80, 8, 938 Publication Date:August 1, 2003
  19. "Chemical elements listed by ionization energy". lenntech.com. Lenntech BV. 2018. Retrieved December 6, 2020. The elements of the periodic table sorted by ionization energy click on any element's name for further information on chemical properties, environmental data or health effects. This list contains the 118 elements of chemistry.
  20. Boudreaux, K.A. (August 13, 2020) [July 26, 2006]. "The Parts of the Periodic Table". Department of Chemistry and Biochemistry. angelo.edu/faculty/kboudrea/. 2601 W. Avenue N, San Angelo, TX 76909, Texas: Angelo State University. Retrieved December 19, 2020 – via angelo.edu.CS1 maint: location (link)
  21. "18.10: The Group 6A Elements". Chemistry LibreTexts. 2014-07-02. Retrieved 2020-09-20.
  22. "Covalent Radius for all the elements in the Periodic Table". periodictable.com. Retrieved 2020-09-13.
  23. Sikander (December 5, 2015). "Why is ionisation energy of bismuth lower than lead?". chemistry.stackexchange.com. Chemistry Stack Exchange. Retrieved December 5, 2020. Why is ionisation enthalpy of Bismuth less than that of Lead for it just comes after the latter in periodic table?
  24. Ball, Philip (April 21, 2017). "The group 3 dilemma". chemistryworld.com. Burlington House, Piccadilly, London: Chemistry World. Retrieved December 18, 2020 – via Royal Society of Chemistry.
  25. Yirka, Bob (April 9, 2015). "Measurement of first ionization potential of lawrencium reignites debate over periodic table". General Physics. phys.org. Phys Org. Phys.org. Retrieved December 13, 2020. Lawrencium, at this time, appears to have a dumb-bell shape. These new findings create conflicting views on where the element should be placed on the table and has reignited debate on the way the table is structured in general.
  26. Scerri, Eric R.; Parsons, William (March 2017). "What elements belong in group 3 of the periodic table?". www.ionicviper.org. Ionic Viper. Retrieved December 7, 2020. The question of precisely which elements should be placed in group 3 of the periodic table has been debated from time to time with apparently no resolution up to this point.
  27. Sato, T.K.; Asai, M.; Borschevsky, A.; Stora, T.; Sato, N.; et al. (April 9, 2015) [November 27, 2014]. "Measurement of the first ionization potential of lawrencium, element 103". Ibaraki University. Nature. Mito, Ibaraki 310-8512, Japan: Nature.com. 520 (7546): 209–211. doi:10.1038/nature14342. PMID 25855457. S2CID 4384213. Retrieved December 13, 2020 – via Japan Atomic Energy Agency (JAEA).CS1 maint: location (link)
  28. Singh, S.J. (September 9, 1999) [September 1, 1999]. "23". The Sterling Dictionary of Physics (1st ed.). A-59, Okhla Industrial Area, Phase-II, New Delhi-110020, India: Sterling Publishers Pvt. Ltd (published September 1, 1999). p. 122. ISBN 978-8173591242. Retrieved December 19, 2020 – via Skyline University College.CS1 maint: location (link)
  29. Greenwood, N.N.; Earnshaw, A. (December 2, 2012) [January 1, 1984]. "22". In Kemmitt, R.D.W.; Wolsey, W.C. (eds.). Chemistry of the Elements. Chemistry of the Elements (2nd ed.). School of Chemistry, University of Leeds, West Yorkshire, England, United Kingdom: Elsevier Butterworth-Heinemann Ltd. (published November 11, 1997). p. 1342. doi:10.1016/C2009-0-30414-6. ISBN 978-0-08-050109-3. OCLC 1005231772. S2CID 93902610. Retrieved December 19, 2020 – via books.google.com.
  30. Housecroft, C.E.; Sharpe, A.G. (November 1, 1993). Inorganic Chemistry (eBook). Inorganic Chemistry. 3 (15th ed.). Switzerland: Pearson Prentice-Hall. p. 536, 649, 743. doi:10.1021/ed070pA304.1. ISBN 9780273742753. Archived from the original on December 16, 2015. Retrieved December 14, 2020 – via University of Basel.
  31. Cotton, F. Albert; Wilkinson, Geoffrey (1988), Advanced Inorganic Chemistry (5th ed.), New York: Wiley-Interscience, pp. 776, 955, ISBN 0-471-84997-9
  32. Jolly, W.L. (1991) [April 1, 1985]. "14". In Billo, E.J.; Amar, J.S.; Zlotnick, Stephen; Hess, C.; Logan, F. (eds.). Modern Inorganic Chemistry. Modern Inorganic Chemistry (2nd ed.). New York City, New York, USA: McGraw-Hill, Inc. (published February 16, 2010). p. 22. doi:10.1021/ed062pA137.1. ISBN 9780070327689. OCLC 22861992. Archived from the original on June 27, 2019. Retrieved December 19, 2020 – via University of Michigan.
  33. "Patterns and trends in the periodic table - Periodicity - Higher Chemistry Revision". BBC Bitesize. Retrieved 2020-09-20.
  34. "Ionization Energies". Chemistry LibreTexts. 2013-10-03. Retrieved 2020-09-20.
  35. "IYPT 2019 Elements 087: Francium: Not the most reactive Group 1 element". Compound Interest. 2019-11-06. Retrieved 2020-09-20.
  36. "The Group 14 elements". Chemistry Nexus. 2015-12-02. Retrieved 2020-09-13.
  37. "Hafnium". gordonengland.co.uk. Gordon England. 2020. Retrieved December 7, 2020. ...Atomic Radius 159 pm...
  38. "Zirconium (Element) - Atomic Radius". pubchem.ncbi.nlm.nih.gov. PubChem®. Retrieved December 8, 2020. 155 pm (Empirical)
  39. Slater, J.C (1964). "Atomic Radii in Crystals". The Journal of Chemical Physics. AIP. 41 (10): 3199–3205. doi:10.1063/1.1725697. Retrieved December 8, 2020.
  40. "WebElements Periodic Table » Titanium » radii of atoms and ions". www.webelements.com. Retrieved 2020-09-20.
  41. Straka, J. "Periodic Table of the Elements: Zirconium - Electronic configuration". www.tabulka.cz. Retrieved 2020-09-20.
  42. "Tantalum | chemical element". Encyclopedia Britannica. Retrieved 2020-09-20.
  43. Langård, Sverre (2015), "Chromium, Molybdenum, and Tungsten", Patty's Toxicology, American Cancer Society, doi:10.1002/0471435139.tox038, ISBN 978-0-471-12547-1, retrieved 2020-09-20
  44. Lang, Peter F.; Smith, Barry C. (August 2003). "Ionization Energies of Atoms and Atomic Ions" (PDF). Journal of Chemical Education. 80 (8): 938. Bibcode:2003JChEd..80..938L. doi:10.1021/ed080p938. ISSN 0021-9584.
  45. "Orbitals, Electron Clouds, Probabilities, and Energies". chem.libretexts.org. UC Davis ChemWiki. May 23, 2019. Retrieved November 2, 2020.
  46. "Quantum numbers and orbitals- The quantum mechanical model of the atom". Khan Academy. Retrieved November 2, 2020.
  47. "Background Reading for Ionization Energy". shodor.org. The Shodor Education Foundation, Inc. 2000. Retrieved November 15, 2020. ... The second method is called Koopman's Theory. This method involves the HOMO.
  48. "The difference between a vertical ionization energy and adiabatic ionization energy". Computational Chemistry Comparison and Benchmark Database. National Institute of Standards and Technology.
  49. Murphy, Andrew; Wong, Monica (2019). "Electron binding energy". radiopaedia.org. Radiopaedia. Retrieved December 7, 2020. The electron binding energy is the minimum energy that is required to remove an electron from an atom
  50. Kittel, Charles (January 1, 1996) [1953]. "6". In Zainab, R.; Du, D.; Tanner, B.K.; Chambers, R.G. (eds.). Introduction to Solid State Physics. Physics Today. 7. New York, USA: John Wiley & Sons, Inc. (published 1995). pp. 18–19. Bibcode:1969Natur.224..983C. doi:10.1063/1.3061720. ISBN 978-0-471-11181-8. LCCN 95-018445. OCLC 263625446. S2CID 121571376. Archived from the original on January 13, 2017. Retrieved December 18, 2020 – via worldcat.org.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.