15 (number)
15 (fifteen) is a number, numeral, and glyph. It is the natural number following 14 and preceding 16.
| ||||
|---|---|---|---|---|
[[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] | ||||
| Cardinal | fifteen | |||
| Ordinal | 15th (fifteenth) | |||
| Numeral system | pentadecimal | |||
| Factorization | 3 × 5 | |||
| Divisors | 1, 3, 5, 15 | |||
| Greek numeral | ΙΕ´ | |||
| Roman numeral | XV | |||
| Binary | 11112 | |||
| Ternary | 1203 | |||
| Octal | 178 | |||
| Duodecimal | 1312 | |||
| Hexadecimal | F16 | |||
| Hebrew | ט"ו (Tet Vav) | |||
Mathematics
15 is:
- a lucky number.
- a triangular number.
- a hexagonal number.[1]
- a pentatope number.[2]
- along with 13, one of two numbers within the teen numerical range (13-19) not to use a single-digit number preceding the teen suffix; instead, it uses the adjective form of five (fifth) as the predecessor of said teen suffix.
- the 5th bell number (i.e. the number of partitions for a set of size 4).[3]
- a composite number; its proper divisors being 1, 3 and 5.
- the number of supersingular primes.
- a repdigit in binary (1111) and quaternary (33). In hexadecimal, as well as all higher bases, 15 is represented as F.
- the smallest number that can be factorized using Shor's quantum algorithm.
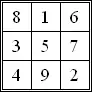
- the magic constant of the unique order-3 normal magic square:
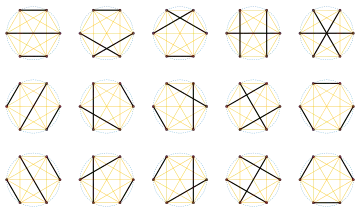
There are 15 perfect matchings of the complete graph K6 and 15 rooted binary trees with four labeled leaves, both of these being among the types of object counted by double factorials. With only two exceptions, all prime quadruplets enclose a multiple of 15, with 15 itself being enclosed by the quadruplet (11, 13, 17, 19).
Because 15 is the product of distinct Fermat primes, 3 and 5, a regular polygon with 15 sides is constructible with compass and unmarked straightedge, and cos π/15 is expressible in terms of square roots (see here).
If a positive definite quadratic form with integer matrix represents all positive integers up to 15, then it represents all positive integers via the 15 and 290 theorems.
15 contains the decimal digits 1 and 5, and is the result of adding together the integers from 1 to 5 (1 + 2 + 3 + 4 + 5 = 15)
Science
- The atomic number of phosphorus.
- Group 15 of the periodic table are sometimes known as the pnictogens.
Religion
Sunnism
The Hanbali Sunni madhab states that the age of fifteen of a solar or lunar calendar is when one's taklif (obligation or responsibility) begins and is the stage whereby one has his deeds recorded.[4]
Judaism
- In the Hebrew numbering system, the number 15 is not written according to the usual method, with the letters that represent "10" and "5" (י-ה, yodh and heh), because those spell out one of the Jewish names of God. Instead, the date is written with the letters representing "9" and "6" (ט-ו, teth and vav)
- Passover begins on the 15th day of the Hebrew month of Nisan
- Sukkot begins on the 15th day of the Hebrew month of Tishrei
- Tu Bishvat is a Jewish holiday occurring on the 15th of the Hebrew month of Shevat
Modern use
15 is designated as an emergency number in Pakistan , for mobile phones, similar to the international GSM emergency number 112; if 112 is used in Pakistan, then the call is routed to 15. 112 can be used in an emergency even if the phone is locked and does not have a SIM card in it.
Sports
- In all four of the major Gaelic games—hurling, Gaelic football for men and women, and camogie—each team has 15 players on the field at any given time.
- In both major forms of gridiron football (American and Canadian), standard games are played in 15-minute quarters.
- In rugby union:
- Each team has 15 players on the field at any given time.
- The jersey number 15 is worn by the starting fullback.
- In tennis, the number 15 represents the first point gained in a game.
- In NCAA Division I women's college basketball, teams are allowed to provide scholarships to no more than 15 players at any given time.
- In Australian rules football, games in the AFL Women's league consist of 15-minute quarters.
Age 15
- The age of a quinceañera, a Latina girl celebrating her 15th birthday. Thus, Spanish bingo callers might refer to the number 15 as la niña bonita (the beautiful girl).
- The age for obtaining a driver's (or learner's) permit in certain jurisdictions, including some, where the legal age for a driver's license is 16.
- In the UK a minor can be sent to prison to await trial at the age of 15.
- In some countries it is the legal age for sexual intercourse.[5]
- In the UK it is the legal age to watch a BBFC "15" rated film in a cinema or purchase a "15" rated film or video game.
- The drinking age in Ethiopia.
- The age of majority in Indonesia, Myanmar, and Yemen.
Music
- "Fifteen" is a song composed by Taylor Swift on her album Fearless
In other fields

Fifteen is:
- The 15 puzzle.
- The number of minutes in one quarter of an hour; 15 minutes past or before an hour is often known as quarter past and quarter to, respectively.
See also
References
- "Sloane's A000384 : Hexagonal numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-01.
- "Sloane's A000332 : Binomial coefficient binomial(n,4) = n*(n-1)*(n-2)*(n-3)/24". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-01.
- "Sloane's A000110 : Bell or exponential numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-01.
- Spevack, Aaron (2011). Ghazali on the Principles of Islamic Spiritualit. p. 50.
- Worldwide Ages of Consent. Avert.org. Retrieved on 2011-11-01.
Further reading
- Wells, D. The Penguin Dictionary of Curious and Interesting Numbers London: Penguin Group. (1987): 91–93
External links
| Look up 15 in Wiktionary, the free dictionary. |
- Clewett, James. "15: f in hexadecimal". Numberphile. Brady Haran. Archived from the original on 2013-05-16. Retrieved 2013-04-02. – discussing hexadecimals
- Bowley, Roger. "15: Bumfit". Numberphile. Brady Haran. Archived from the original on 2013-05-16. Retrieved 2013-04-01. – discussing the Celtic number as used in Lincolnshire