Infinite-order apeirogonal tiling

In geometry, the infinite-order apeirogonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {∞,∞}, which means it has countably infinitely many apeirogons around all its ideal vertices.

Infinite-order apeirogonal tiling
Poincaré disk model of the hyperbolic plane
Type	Hyperbolic regular tiling
Vertex configuration	∞^∞
Schläfli symbol	{∞,∞}
Wythoff symbol	∞ 2 ∞ ∞ \| ∞
Coxeter diagram
Symmetry group	[∞,∞], (∞∞2) [(∞,∞,∞)], (∞∞∞)
Dual	self-dual
Properties	Vertex-transitive, edge-transitive, face-transitive

Symmetry

This tiling represents the fundamental domains of *∞^∞ symmetry.

This tiling can also be alternately colored in the [(∞,∞,∞)] symmetry from 3 generator positions.

Domains	0	1	2
symmetry: [(∞,∞,∞)]	t₀{(∞,∞,∞)}	t₁{(∞,∞,∞)}	t₂{(∞,∞,∞)}

The union of this tiling and its dual can be seen as orthogonal red and blue lines here, and combined define the lines of a *2∞2∞ fundamental domain.

a{∞,∞} or

=

∪

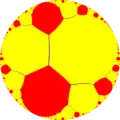
Paracompact uniform tilings in [∞,∞] family
= =	= =	= =	= =	= =	=	=

{∞,∞}	t{∞,∞}	r{∞,∞}	2t{∞,∞}=t{∞,∞}	2r{∞,∞}={∞,∞}	rr{∞,∞}	tr{∞,∞}
Dual tilings


V∞^∞	V∞.∞.∞	V(∞.∞)²	V∞.∞.∞	V∞^∞	V4.∞.4.∞	V4.4.∞
Alternations
[1⁺,∞,∞] (*∞∞2)	[∞⁺,∞] (∞*∞)	[∞,1⁺,∞] (*∞∞∞∞)	[∞,∞⁺] (∞*∞)	[∞,∞,1⁺] (*∞∞2)	[(∞,∞,2⁺)] (2*∞∞)	[∞,∞]⁺ (2∞∞)


h{∞,∞}	s{∞,∞}	hr{∞,∞}	s{∞,∞}	h₂{∞,∞}	hrr{∞,∞}	sr{∞,∞}
Alternation duals


V(∞.∞)^∞	V(3.∞)³	V(∞.4)⁴	V(3.∞)³	V∞^∞	V(4.∞.4)²	V3.3.∞.3.∞

Paracompact uniform tilings in [(∞,∞,∞)] family



(∞,∞,∞) h{∞,∞}	r(∞,∞,∞) h₂{∞,∞}	(∞,∞,∞) h{∞,∞}	r(∞,∞,∞) h₂{∞,∞}	(∞,∞,∞) h{∞,∞}	r(∞,∞,∞) r{∞,∞}	t(∞,∞,∞) t{∞,∞}
Dual tilings

V∞^∞	V∞.∞.∞.∞	V∞^∞	V∞.∞.∞.∞	V∞^∞	V∞.∞.∞.∞	V∞.∞.∞
Alternations
[(1⁺,∞,∞,∞)] (*∞∞∞∞)	[∞⁺,∞,∞)] (∞*∞)	[∞,1⁺,∞,∞)] (*∞∞∞∞)	[∞,∞⁺,∞)] (∞*∞)	[(∞,∞,∞,1⁺)] (*∞∞∞∞)	[(∞,∞,∞⁺)] (∞*∞)	[∞,∞,∞)]⁺ (∞∞∞)


Alternation duals

V(∞.∞)^∞	V(∞.4)⁴	V(∞.∞)^∞	V(∞.4)⁴	V(∞.∞)^∞	V(∞.4)⁴	V3.∞.3.∞.3.∞

John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
"Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.